Sample preparation
Samples with various Fe thicknesses tFe are grown by molecular-beam epitaxy (MBE). First, a GaAs buffer layer of 100ânm is grown in a IIIâV MBE. After that the substrate (semi-insulating wafer, which has a resistivity Ï between 1.72âÃâ108âΩâcm and 2.16âÃâ108âΩâcm) is transferred to a metal MBE without breaking the vacuum for the growth of the metal layers. For a better comparison of the physical properties of different samples, various Fe thicknesses are grown on a single two-inch wafer by stepping the main shadow shutter of the metal MBE. After the growth of the step-wedged Fe film, 1.5-nm Al/6-nm Pt layers are deposited on the whole wafer. Sharp reflection high-energy electron diffraction patterns have been observed after the growth of each layer (Supplementary Note 1), which indicate the epitaxial growth mode as well as good surface (interface) flatness. High-resolution transmission electron microscopy measurements (Supplementary Note 1) show that (1) all the layers are crystalline and (2) there is diffusion of Al into Pt but no significant AlâFe and PtâFe interdiffusion. Therefore, the magnetic proximity effect between Fe and Pt is reduced. The intermixed PtâAl alloy can be a good spin current generator. Previous work49 has shown that alloying Pt with Al enhances the spin-torque efficiency.
Device fabrication
First, Pt/Al/Fe stripes with a dimension of 4âμmâÃâ20âμm and with the long side along the [110] and [100] orientations are defined by a mask-free writer and Ar-etching. After that, contact pads for the application of the d.c. current, which are made from 3-nm Ti and 50ânm Au, are prepared by evaporation and lift-off. Then, a 70-nm Al2O3 layer is deposited by atomic layer deposition to electrically isolate the d.c. contacts and the coplanar waveguide (CPW). Finally, the CPW consisting of 5ânm Ti and 150ânm Au is fabricated by evaporation, and the Fe/Al/Pt stripes are located in the gap between the signal line and ground line of the CPW (Fig. 2a). During the fabrication, the highest baking temperature is 110â°C. The CPW is designed to match the radiofrequency network that has an impedance of 50âΩ. The width of the signal line and the gap are 50âμm and 30âμm, respectively. Magnetization dynamics of Fe are excited by out-of-plane Oersted field induced by the radiofrequency microwave currents flowing in the signal and ground lines.
FMR measurements
The FMR method is used in this study for several reasons: (1) FMR has a higher sensitivity than static magnetization measurements. (2) The FMR method, together with angle and frequency-dependent measurements, is a standard way to quantify the effective magnetization, magnetic anisotropies and Gilbert damping. (3) Damping-like and field-like torques can be determined simultaneously in a single experiment, and thus we can establish a connection between damping-like torque and the modification of magnetic anisotropies. (4) The Joule heating effect, which also alters the magnetic properties of Fe, can be easily excluded from the I dependence of HR.
The FMR spectra are measured optically by time-resolved magneto-optical Kerr microscopy; a pulse train of a Ti:sapphire laser (repetition rate of 80âMHz and pulse width of 150âfs) with a wavelength of 800ânm is phase-locked to a microwave current. A phase shifter is used to adjust the phase between the laser pulse train and microwave, and the phase is kept constant during the measurement. The polar Kerr signal at a certain phase, VKerr, is detected by a lock-in amplifier by phase modulating the microwave current at a frequency of 6.6âkHz. The VKerr signal is measured by sweeping the external magnetic field, and the magnetic field can be rotated in-plane by 360°. A Keithley 2400 device is used as the d.c. current source for linewidth and resonance field modifications. All measurements are performed at room temperature.
The FMR spectra are well fitted by combining a symmetric (Lsymâ=âÎH2/[4(HâââHR)2â+âÎH2]) and an anti-symmetric Lorentzian (La-symâ=ââ4ÎH(HâââHR)/[4(HâââHR)2â+âÎH2]), VKerrâ=âVsymLsymâ+âVa-symLa-symâ+âVoffset, where HR is the resonance field, ÎH is the full width at half maximum, Voffset is the offset voltage, and Vsym (Va-sym) is the magnitude of the symmetric (anti-symmetric) component of VKerr. It is worth mentioning that, by analysing the position of HR, we have also confirmed that the application of the charge currents does not have a detrimental effect on the magnetic properties of the Fe films (Supplementary Note 2).
Magnetic anisotropies in Pt/Al/Fe/GaAs multilayers
A typical in-plane magnetic field angle ÏH dependence of the resonance field HR for tFeâ=â1.2ânm measured at fâ=â13âGHz is shown in Extended Data Fig. 2a. The sample shows typical in-plane uniaxial anisotropy with two-fold symmetry, that is, a magnetically HA for ÏHâ=ââ45° and 135° (\(\langle \bar{1}10\rangle \) orientations) and a magnetically EA for ÏHâ=â45° and 225° (â¨110â© orientations), which originates from the anisotropic bonding at the Fe/GaAs interface33. To quantify the magnitude of the anisotropies, we further measure the f dependence of HR both along the EA and the HA (Extended Data Fig. 2b). Both the angle and frequency dependence of HR are fitted according to34,50
$${\left(\frac{2{\rm{\pi }}f}{\gamma }\right)}^{2}={\mu }_{0}^{2}{H}_{1}^{\text{R}}{H}_{2}^{\text{R}},$$
(5)
with \({H}_{1}^{\text{R}}\)â=âHRâcos(ÏâââÏH)â+âHKâ+âHB(3â+âcosâ4Ï)/4âââHUâsin2(Ïâââ45°) and \({H}_{2}^{\text{R}}\)â=âHRâcos(ÏâââÏH)â+ âHBâcosâ4ÏâââHUâsinâ2Ï. Here γ (=âgμB/ħ) is the gyromagnetic ratio, g is the Landé g-factor, μB is the Bohr magneton, ħ is the reduced Planck constant, HK (=âMâââHâ¥) is the effective demagnetization magnetic anisotropy field, including the perpendicular magnetic anisotropy field Hâ¥, HB is the biaxial magnetic anisotropy field along the â¨100â© orientations, HU is the in-plane UMA field along â¨110â© orientations and Ï is the in-plane angle of magnetization as defined in Extended Data Fig. 1. The magnitude of Ï is obtained by the equilibrium condition
$${H}_{{\rm{R}}}\,\sin (\varphi -{\varphi }_{H})+({H}_{{\rm{B}}}/4)\sin 4\varphi +({H}_{{\rm{U}}}/2)\cos 2\varphi =0.$$
(6)
It can be checked that Ïâ=âÏH holds when H is along â¨110â© and \(\langle \bar{1}10\rangle \) orientations. From the fits of HR, the magnitude of the magnetic anisotropy fields HA (HAâ=âHK,âHB,âHU) for each tFe is obtained, and their dependences on inverse Fe thickness \({t}_{\text{Fe}}^{{-}1}\), together with the results obtained from the AlOx/Fe/GaAs samples, are shown in Extended Data Fig. 2c. The results show that the Pt/Al/Fe/GaAs samples have virtually identical magnetic anisotropies as the AlOx/Fe/GaAs samples, and introducing the Pt/Al layer neither enhances the magnetization leading to an increase in HK nor generates a perpendicular anisotropy leading to a decrease in HK. By comparing the values of HK and M, we confirm that the main contribution to HK stems from the magnetization due to the demagnetization field. For both sample series, HK and HB decrease as tFe decreases because of the reduction of the magnetization as tFe decreases, and both of them scale linearly with \({t}_{\text{Fe}}^{{-}1}\). The intercept (about 2,220âmT) of the \({H}_{{\rm{K}}}-{t}_{\text{Fe}}^{-1}\) trace corresponds to the saturation magnetization of bulk Fe, and the intercept (around 45âmT) of the \({H}_{{\rm{B}}}-{t}_{\text{Fe}}^{-1}\) trace corresponds to the biaxial anisotropy of bulk Fe. In contrast to HK and HB, HU shows a linear dependence on \({t}_{\text{Fe}}^{{-}1}\) with a zero intercept, indicative of the interfacial origin of HU.
Effective mixing conductance in Pt/Al/Fe/GaAs multilayers
Extended Data Fig. 3a,b shows the ÏH dependence and f dependence, respectively, of linewidth ÎH for tFeâ=â1.2ânm. The magnitude of ÎH varies strongly with ÏH because of the presence of in-plane anisotropy, and the dependencies of ÎH on f along both EA and HA show linear behaviour. Both the angular and frequency dependence of ÎH can be well fitted by51
$$\Delta H=\Delta [\text{Im}(\chi )]+\Delta {H}_{0}=\Delta \left[\frac{\alpha \sqrt{{H}_{1}^{\text{R}}{H}_{2}^{\text{R}}}({H}_{1}{H}_{1}+{H}_{1}^{\text{R}}{H}_{2}^{\text{R}})M}{{({H}_{1}{H}_{2}-{H}_{1}^{\text{R}}{H}_{2}^{\text{R}})}^{2}+{\alpha }^{2}{H}_{1}^{\text{R}}{H}_{2}^{\text{R}}{({H}_{1}+{H}_{2})}^{2}}\right]+\Delta {H}_{0},$$
(7)
where Î[Im(Ï)] is the linewidth of the imaginary part of the dynamic magnetic susceptibility Im(Ï), H1 and H2 are defined in equation (5) for arbitrary H values, and ÎH0 is the residual linewidth (zero-frequency intercept). As the angular trace can be well fitted by using a damping value of 0.0078, there is no need to consider other extrinsic effects (that is, inhomogeneity and/or two-magnon scattering) contributing to ÎH. It is worth mentioning that the angular trace gives a slightly higher α value because ÎH0, which also depends on ÏH, is not considered in the fit. In this case, the frequency dependence of linewidth gives more reliable damping values (Extended Data Fig. 3b). Extended Data Fig. 3c compares the magnitude of damping for Pt/Al/Fe/GaAs and AlOx/Fe/GaAs samples. For both sample series, the Gilbert damping increases as tFe decreases and a linear dependence of α on \({t}_{\text{Fe}}^{{-}1}\) is observed. The enhancement of α is because of the spin pumping effect, which is given by52,53
$$\alpha ={\alpha }_{0}\,+\,{g}_{{\rm{eff}}}^{\uparrow \downarrow }\frac{\gamma \hbar }{4{\rm{\pi }}M}{t}_{{\rm{Fe}}}^{-1},$$
(8)
where α0 is the intrinsic damping of pure bulk Fe and \({g}_{{\rm{eff}}}^{\uparrow \downarrow }\) is the effective spin mixing conductance quantifying the spin pumping efficiency. By using μ0Mâ=â2.2âT and γâ=â1.80âÃâ1011 radâsâ1âTâ1, the magnitude of \({g}_{{\rm{eff}}}^{\uparrow \downarrow }\) for Pt/Al/Fe/GaAs is determined to be 4.6âÃâ1018âmâ2, and \({g}_{{\rm{eff}}}^{\uparrow \downarrow }\) at the Fe/GaAs interface is determined to be 1.9âÃâ1018âmâ2. Therefore, by subtracting these two values, the magnitude of \({g}_{{\rm{eff}}}^{\uparrow \downarrow }\) at Pt/Al/Fe interface is determined to be 2.7âÃâ1018âmâ2. The spin transparency Tint of the Pt/Al/Fe interface is given by ref. 53
$${T}_{{\rm{int}}}=\frac{2{e}^{2}}{h}\frac{{g}_{{\rm{eff}}}^{\uparrow \downarrow }}{{G}_{{\rm{Pt}}}}$$
(9)
where 2e2/h is the conductance quantum, GPt [=â1/(Ïxxλs)] is the spin conductance of Pt, Ïxx is the resistivity and λs is the spin diffusion length. By using λsâ=â4ânm and an averaged Ïxxâ=â40âμΩâcm, Tintâ=â0.21 is determined. We note that the magnitude of \({g}_{{\rm{eff}}}^{\uparrow \downarrow }\) at the Pt/Al/Fe interface is about one order of magnitude smaller than the experimental values found at heavy metal/ultrathin ferromagnet interfaces54, but very close to the value obtained by the first-principles calculations55. The previously overestimated \({g}_{{\rm{eff}}}^{\uparrow \downarrow }\) and thus Tint at heavy metal/ultrathin ferromagnet interfaces is probably because the enhancement of α by two-magnon scattering56 as well as by the magnetic proximity effect (see Supplementary Note 3) is not properly excluded. Moreover, the obtained α0 values for Pt/Al/Fe/GaAs (α0â=â0.0039) and AlOx/Fe/GaAs (α0â=â0.0033) slightly differ; the reason is unclear to us, but might be because of a small error in the Fe thickness, which is hard to be determined accurately in the ultrathin regime.
Theory of the modulation of the linewidth
To model the modulation of the FMR linewidth by the application of d.c. current, the LandauâLifshitzâGilbert equation with damping-like spin-torque term is considered18,35,
$$\frac{{\rm{d}}{\bf{M}}}{{\rm{d}}t}=-\gamma {\bf{M}}\times {\mu }_{0}{{\bf{H}}}_{{\rm{e}}{\rm{f}}{\rm{f}}}+\frac{\alpha }{M}{\bf{M}}\times \frac{{\rm{d}}{\bf{M}}}{{\rm{d}}t}-\frac{\gamma {\mu }_{0}{h}_{{\rm{D}}{\rm{L}}}}{M}{\bf{M}}\times {\bf{M}}\times {\boldsymbol{\sigma }}.$$
(10)
The terms on the right side of equation (10) correspond to the precession torque, the damping torque and the damping-like spin torque induced by the spin current. Here Ï is the spin polarization unit vector, and hDL is the effective anti-damping-like magnetic field. The effective magnetic field Heff, containing both external and internal fields, is expressed in terms of the free energy density F, which can be obtained as
$${{\bf{H}}}_{\text{eff}}=-\frac{1}{{\mu }_{0}}\frac{\partial F}{\partial {\bf{M}}}.$$
(11)
For single-crystalline Fe films grown on GaAs(001) substrates with in-plane magnetic anisotropies, F is given by34,58
$$\,F=\frac{{\mu }_{0}M}{2}\left\{-2H[\cos \theta \cos {\theta }_{H}+\sin \theta \sin {\theta }_{\text{H}}\cos (\varphi -{\varphi }_{H})]+{H}_{\text{K}}{\cos }^{2}\theta -\frac{{H}_{\text{B}}}{2}{\sin }^{4}\theta \frac{3+\cos 4\varphi }{4}-{H}_{\text{U}}{\sin }^{2}\theta {\sin }^{2}\left(\varphi -\frac{{\rm{\pi }}}{4}\right)\right\}.$$
(12)
Bringing equations (11) and (12) into equation (10), the time-resolved magnetization dynamics for current flowing along the [110] orientation (that is, Ï â¥â\([\bar{1}10]\)) is obtained as
$$\left\{\begin{array}{l}\frac{\partial \varphi }{\partial t}=\frac{\gamma {\mu }_{0}}{\left(1+{\alpha }^{2}\right)M\sin \theta }\left(\frac{\partial F}{\partial \theta }-\frac{\alpha }{\sin \theta }\frac{\partial F}{\partial \varphi }\right)+\frac{\gamma {\mu }_{0}{h}_{{DL}}}{\left(1+{\alpha }^{2}\right)\sin \theta }\frac{\sqrt{2}}{2}\left[\alpha \cos \theta \left(\sin \varphi -\cos \varphi \right)+\cos \varphi +\sin \varphi \right]\\ \frac{\partial \theta }{\partial t}=\frac{\gamma {\mu }_{0}}{M\sin \theta }\left(\frac{{\alpha }^{2}}{1+{\alpha }^{2}}-1\right)\frac{\partial F}{\partial \varphi }-\frac{\alpha }{1+{\alpha }^{2}}\frac{\gamma {\mu }_{0}}{M}\frac{\partial F}{\partial \theta }+\left(1+\frac{{\alpha }^{2}}{1+{\alpha }^{2}}\right)\gamma {\mu }_{0}{h}_{{DL}}\frac{\sqrt{2}}{2}\cos \theta \left(\sin \varphi -\cos \varphi \right)+\frac{\alpha }{1+{\alpha }^{2}}\gamma {\mu }_{0}{h}_{{DL}}\frac{\sqrt{2}}{2}\left(\cos \varphi +\sin \varphi \right)\end{array}\right.$$
(13)
Similarly, for the current flowing along the [100]-orientation (that is, Ï â¥â[010]), we have
$$\left\{\begin{array}{l}\frac{\partial \varphi }{\partial t}=\frac{\gamma {\mu }_{0}}{\left(1+{\alpha }^{2}\right)M\sin \theta }\left(\frac{\partial F}{\partial \theta }-\frac{\alpha }{\sin \theta }\frac{\partial F}{\partial \varphi }\right)+\frac{\gamma {\mu }_{0}{h}_{{DL}}}{\left(1+{\alpha }^{2}\right)\sin \theta }\left(\alpha \cos \theta \sin \varphi +\cos \varphi \right)\\ \frac{\partial \theta }{\partial t}=\frac{\gamma {\mu }_{0}}{M\sin \theta }\left(\frac{{\alpha }^{2}}{1+{\alpha }^{2}}-1\right)\frac{\partial F}{\partial \varphi }-\frac{\alpha }{1+{\alpha }^{2}}\frac{\gamma {\mu }_{0}}{M}\frac{\partial F}{\partial \theta }-\gamma {\mu }_{0}{h}_{{DL}}\left[\frac{{\alpha }^{2}}{1+{\alpha }^{2}}\left(\alpha \cos \theta \sin \varphi +\cos \varphi \right)-\cos \theta \sin \varphi \right]\end{array}.\right.$$
(14)
The time dependence of Ï(t), θ(t) and then m(t) can be readily obtained from equations (13) and (14), and Extended Data Fig. 4a shows an example of the time-dependent mz by using μ0Hâ=â101âmT, μ0HKâ=â1,350âmT, μ0HUâ=â128âmT, μ0HBâ=â10âmT, αâ=â0.0063 and μ0HDLâ=â0. The damped oscillating dynamic magnetization can be well fitted by
$${m}_{z}(t)=A{\text{e}}^{-t/\tau }\cos (2{\rm{\pi }}ft+\phi )$$
(15)
where A is the amplitude, Ï is the magnetization relaxation time and Ï is the phase shift. The connection between Ï and ÎH is given by
$$\Delta H=\frac{1}{2{\rm{\pi }}}\left|\frac{{\rm{d}}{H}_{\text{R}}}{{\rm{d}}f}\right|\frac{1}{\tau }$$
(16)
where dHR/df can be readily obtained from equation (5). We confirm the validity of the above method in Extended Data Fig. 4b by showing that the angle dependence of ÎH obtained from the time domain (equation (16)) at hDLâ=â0 is identical to the linewidth obtained by the dynamic susceptibility in the magnetic field domain (equation (7)).
Having obtained the linewidth for Iâ=â0, the next step is to calculate the influence of the linewidth by spinâorbit torque. The magnitude of hDL is given by
$${\mu }_{0}{h}_{\text{DL}}=\frac{\hbar }{2e}\frac{\xi }{M{t}_{\text{Fe}}}{j}_{\text{Pt}}$$
(17)
where ξ is the effective damping-like torque efficiency and jPt is the current density in Pt. For the Pt/Al/Fe multilayer, jPt is determined by the parallel resistor model
$${j}_{\text{Pt}}=\frac{{t}_{\text{Pt}}\,{\rho }_{\text{Al}}{\rho }_{\text{Fe}}}{{t}_{\text{Pt}}\,{\rho }_{\text{Al}}{\rho }_{\text{Fe}}+{t}_{\text{Al}}{\rho }_{\text{Pt}}{\rho }_{\text{Fe}}+{t}_{\text{Fe}}{\rho }_{\text{Pt}}{\rho }_{\text{Al}}}\frac{I}{w{t}_{\text{Pt}}}$$
(18)
where ÏPt (=â40âμΩâcm), ÏAl (=â10âμΩâcm) and ÏFe (=â50âμΩâcm) are the resistivities of the Pt, Al and Fe layers, respectively; tPt, tAl and tFe are the thicknesses of the Pt, Al and Fe layers, respectively; I is the d.c. current; and w is the width of the device. Plugging equations (17) and (18) into equations (13) and (14), the I dependence of ÎH can be obtained. An example is shown in Extended Data Fig. 4c, which shows a linear ÎHâI relationship. From the linear fit (equation (1) in the main text), we obtain the modulation amplitude of ÎH, that is, d(ÎH)/dI. Extended Data Fig. 4d presents the calculated d(ÎH)/dI as a function of the magnetic field angle, which shows a strong variation around the HA.
To reproduce the experimental data as shown in Fig. 1f in the main text, the magnitude of the magnetic anisotropies and the damping parameter obtained in Extended Data Fig. 3 as well as ξâ=â0.06 are used. Note that the distinctive presence of robust UMA at the Fe/GaAs interface significantly alters the angular dependence of d(ÎH)/dI. This deviation is remarkable when compared with the sinÏIâH dependence of d(ÎH)/dI as observed in polycrystalline samples, such as Pt/Py (refs.â57,58).
To understand the strong deviation of d(ÎH)/dI around the HA, we plot the in-plane angular dependence of F in Extended Data Fig. 5 for θâ=âθHâ=â90°, that is,
$$F=\frac{{\mu }_{0}M}{2}\left[-2{H}_{\text{R}}\cos (\varphi -{\varphi }_{H})-\frac{{H}_{\text{B}}}{2}\frac{3+\cos 4\varphi }{4}-{H}_{\text{U}}{\sin }^{2}\left(\varphi -\frac{{\rm{\pi }}}{4}\right)\right].$$
(19)
It shows that, around the HA (approximately ±15°), the magnetic potential barrier completely vanishes and \(\frac{\partial F}{\partial \varphi }=0\) and \(\frac{{\partial }^{2}F}{\partial \varphi } < 0\) hold. This indicates that the net static torques induced by internal and external magnetic fields acting on the magnetization cancel and the magnetization has a large cone angle for precession59. Consequently, the magnetization behaves freely with no constraints in the vicinity of the HA, and the low stiffness allows larger d(ÎH)/dI values induced by spin current60. If there are no in-plane magnetic anisotropies, the free energy is constant and is independent of the angle, the magnetization always follows the direction of the applied magnetic field and has the same stiffness at each position. Therefore, the modulation shows no deviation around the HA.
Frequency dependence of the linewidth modulation
Extended Data Fig. 6a shows the frequency dependence of the modulation of linewidth d(ÎH)/dI for tFeâ=â2.8ânm and 1.2ânm, in which the current flows along the [100] orientation. For both samples, the modulation changes polarity as the direction of M is changed by 180°. The modulation amplitude increases quasi-linearly with frequency, and the experimental results can be also reproduced by equation (14) using ξâ=â0.06, consistent with the angular modulation shown in Fig. 2f. For H along the â¨110â© and \(\langle \bar{1}10\rangle \) orientations, the frequency and the Fe thickness dependence of linewidth modulation is approximately given by24
$$\frac{\text{d}({\mu }_{0}\Delta H)}{\text{d}(I)}=2\frac{2{\rm{\pi }}f}{\gamma }\frac{\sin {\varphi }_{I-H}}{{H}_{\text{R}}+{H}_{\text{K}}/2}\frac{\hbar }{2e}\frac{\xi }{{Mt}_{\text{Fe}}}\frac{1}{{t}_{\text{Pt}}w},$$
(20)
where ÏIâHâ=â45°, 135°, 225° and 315° as shown by the inset of each panel in Extended Data Fig. 6. The damping-like torque efficiency can be further quantified by the slope s of f-dependence modulation, that is, \(s=\frac{\text{d}[\text{d}(\Delta H)\,/\,\text{d}I]}{\text{d}f}\). Extended Data Fig. 7 shows the absolute value of s values as a function of \({t}_{\text{Fe}}^{{-}1}\). A linear dependence of |s| on \({t}_{\text{Fe}}^{{-}1}\) is observed, which indicates that the damping-like torque is an interfacial effect, originating from the absorption of spin current generated in Pt (ref.â61).
Quantifying the modification of the magnetic anisotropies
In this section, we show our procedure to quantify the modulation of magnetic anisotropies by spin currents. According to equation (5), the f dependencies of HR along the EA (ÏHâ=âÏâ=â45° and 225°) and the HA (ÏHâ=âÏâ=â135° and 315°) are given by equation (3). From the angle and frequency dependencies of HR as shown in Extended Data Fig. 2, μ0HKâ=â1,350âmT, μ0HUâ=â128âmT, μ0HBâ=â10âmT and gâ=â2.05 are determined for tFeâ=â1.2ânm. Extended Data Fig. 8a shows the HR dependence of f for μ0HKâ=â1,350âmT (blue solid line) and μ0HKâ+âÎμ0HKâ=â1,400âmT (red solid line) along the HA calculated by equation (3). To exaggerate the difference, μ0ÎHK of 50âmT is assumed. The shift of the resonance field ÎHR is obtained as ÎHRâ=âHR(HK)âââHR(HKâ+âÎHK), and the frequency dependence of ÎHR is plotted in Extended Data Fig. 8b, which shows a linear behaviour with respect to f between 10âGHz and 20âGHz (in the experimental range), that is, ÎHRâ=âkKf. Note that, to simplify the analysis, the zero-frequency intercept is ignored because the magnitude is much smaller than the intercept induced by ÎHU and ÎHB. The sign of the slope kK is the same as that of ÎHK and its magnitude is proportional to ÎHK, that is, kKâââÎHK. For the EA as shown in Extended Data Fig. 8c,d, the ÎHRâf relationship induced by ÎHK remains the same as for the HA, that is, ÎHRâ=âkKf still holds.
Extended Data Fig. 8e shows the HRdependence of f for μ0HUâ=â128âmT (blue solid line) and μ0HUâ+âμ0ÎHUâ=â178âmT (red solid line) along the HA. As shown in Extended Data Fig. 8f, the shift of the resonance field along the HA is independent of f with a negative intercept, that is, ÎHRâ=ââÎHU. However, for the EA, as shown in Extended Data Fig. 8g,h, the f-dependent ÎHR can be expressed as ÎHRâ=âÎHUâââkUf, which has an opposite slope compared with the ÎHRâf relationships induced by âHK (Extended Data Fig. 8d), that is, kUââââÎHU.
If the modulation is induced by a change in the biaxial anisotropy as shown in Extended Data Fig. 8iâl, ÎHR along both the HA and EA shows a linear dependence on f, which is expressed as ÎHRâ=ââÎHBâ+âkBf, and kBâââÎHB holds.
Extended Data Table 1 summarizes the ÎHRâf relationships both along the EA and HA induced by ÎHK, ÎHU and ÎHB.
As hOe/FL generated by the d.c. current also shifts the resonance field along the EA and HA axes by \(\pm \frac{\sqrt{2}}{2}{h}_{\text{Oe}/\text{FL}}\), where plus corresponds to the [110] (EA) and the \([\bar{1}10]\) (HA) directions, and minus corresponds to the \([\bar{1}\bar{1}0]\) (EA) and the \([1\bar{1}0]\) (HA) directions, the total ÎHR induced by ÎHK, ÎHU and ÎHB along the EA and HA is, respectively, given by equation (4).
Based on equations (4) and (5), the values of ÎHK, ÎHU, ÎHB and hOe/FL for tFeââ¤â2.2ânm are extracted as follows:
-
1.
We consider the results obtained for Hâ ⥠âMââ¥â[110] (EA) and Hââ¥âM/\([1\bar{1}0]\) (HA) as shown in Extended Data Fig. 9a (the same results as shown in Fig. 4 in the main text for Iâ=â1âmA), where the net magnetization is parallel to I. At fâ=â0, equation (4) is reduced to
$$\Delta {H}_{\text{R}}^{\text{EA}}(0)=\Delta {H}_{\text{U}}-\Delta {H}_{\text{B}}+\frac{\sqrt{2}}{2}{h}_{\text{Oe}/\text{FL}}=-0.20\,{\rm{mT}}$$
(21)
$$\Delta {H}_{\text{R}}^{\text{HA}}(0)=-(\Delta {H}_{\text{U}}+\Delta {H}_{\text{B}})-\frac{\sqrt{2}}{2}{h}_{\text{Oe}/\text{FL}}=-0.32\,{\rm{mT}}.$$
(22)
By adding equations (21) and (22), the magnitude of ÎHB is determined to be 0.26âmT, which corresponds to kB of 4âÃâ10â3âmTâGHzâ1 according to equation (3).
-
2.
From Extended Data Fig. 9a, the slope along the HA is determined to be kKâ+âkBâ=â0.025âmTâGHzâ1. Thus, the magnitude of kK is determined by kKâ=â0.025âmTâGHzâ1âââkBâ=â0.021âmTâGHzâ1, which corresponds to ÎHKâ=â2.0âmT according to equation (3).
-
3.
As \(\Delta {H}_{\text{R}}^{\text{EA}}\) is frequency independent, this requires that kU = kKâ+âkBâ=â0.025âmTâGHzâ1, which corresponds ÎHUâ=â2.5âmT.
-
4.
As the magnetization along EA and HA is, respectively, rotated by 180° to the \([\bar{1}\bar{1}0]\) and \([\bar{1}10]\) directions, and the net magnetization is antiparallel to I (Extended Data Fig. 9b), we obtain ÎHBâ=ââ0.26âmT, ÎHKâ=ââ2.0âmT and ÎHUâ=ââ2.5âmT, which are of opposite sign as the results obtained from Extended Data Fig. 9a.
-
5.
Finally, bringing the magnitude of ÎHB and ÎHU back into equations (21) and (22), \(\frac{\sqrt{2}}{2}{h}_{\text{Oe}/\text{FL}}\) is determined to be â2.24âmT. The negative sign of hOe/FL indicates that it is along the \([0\bar{1}0]\) orientation.
Similarly, the corresponding ÎHB, ÎHK and ÎHU values can be determined for tFeâ=â2.2ânm (Extended Data Fig. 10). Extended Data Table 2 summarizes the magnitudes of the magnetic anisotropy modifications as well as the hOe/FL values for all the devices. The enhancement of the field-like torque in thinner samples has been observed in other systems and is probably because of the enhanced BychkovâRashba spinâorbit interaction61,62 and/or the orbital angular momentum (orbital Hall effect and orbital Rashba effect) at the ferromagnetic metal/heavy metal interface62.
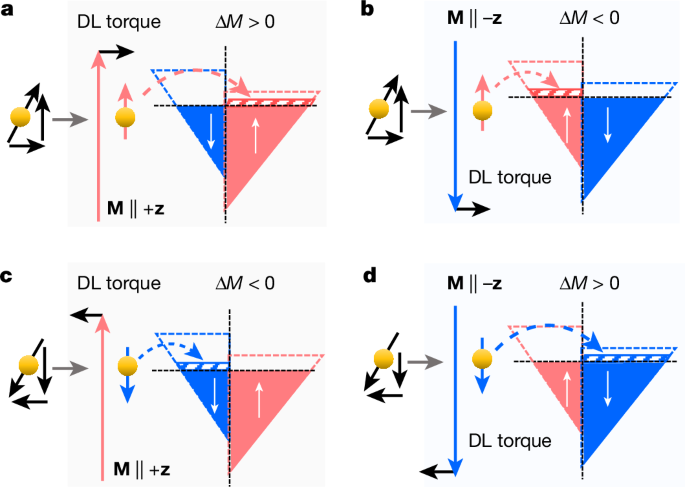
It is worth mentioning that, once the magnetization direction is fixed, ÎHB, ÎHK and ÎHU obtained either from Extended Data Fig. 9a (Extended Data Fig. 10a) or from Extended Data Fig. 9b (Extended Data Fig. 10b) have the same sign (either positive or negative depending on the direction of M). This is consistent with the change in magnetic anisotropies by temperature (Supplementary Fig. 7), which shows that the magnitude of ÎHB, HK and ÎHU increases as the temperature decreases and decreases as the temperature increases. This indicates that the increase in the magnetic anisotropies is dominated by the increase in M as temperature decreases and the decrease in the magnetic anisotropies is dominated by the decrease in M as temperature increases. For the spin current modification demonstrated here, the temperature is not changed but the change in M is induced by populating the electronic bands by the spin current. More interestingly, the new modification method can control the increase or decrease in M simply by the direction of current and/or the direction of magnetization, which is not accessible by other controls.
Alternative interpretation of the experimental results
It is known that the starting point of the FMR analysis is the static magnetic energy landscape, which is related to the magnetic anisotropies. Therefore, it is natural to consider that the modification of magnetic anisotropy accounts for the f-linear dHR/dI curves as observed in the experiment. Although the data analysis discussed in the previous section is self-consistent, there could be alternative interpretations of the data. One possibility could be the current-induced modification of the Landé g-factor of Fe. In magnetic materials, it is known that g is related to the orbital moment μL and the spin moment μS:
$$g=\frac{2{\mu }_{\text{L}}}{{\mu }_{\text{S}}}+2.$$
(23)
A flow of spin and orbital angular momentum induced by charge current could, respectively, modify the orbital and spin moment of Fe by ÎμS and ÎμL, and then a change in the gyromagnetic ratio of Fe is expected. This could, in turn, lead to a shift of FMR resonance fields linearly depending on the frequency. However, if this were the case, an anisotropic modification of g is needed to interpret the data as observed in Extended Data Figs. 9 and 10 (that is, there is sizeable modification along the HA, but no modification along the EA). As we cannot figure out why the modification of g could be anisotropic, we ignore the discussion of the g-factor modification in the main text. We are also open to other possible explanations for the experimental observations.
Estimation of the magnitude of spin transfer electrons
The change in magnetization is attributed to the additional filling of the electronic d-band. The induced filling of the bands in Fe occurs mainly close to the interface and is not homogeneously distributed, as it depends on the spin diffusion length of the spin current in Fe. In other words, the measured modulated magnetic anisotropies are averaged over the whole ferromagnetic film. For simplicity, we neglect the spin current distribution in Fe and assume that it is homogeneously distributed. The spin chemical potential at the interface63 is given by \({u}_{\text{s}}^{0}=2e\lambda \xi E\tanh \left(\frac{{t}_{\text{Pt}}}{2\lambda }\right)\), where e is the elementary charge, λ is the spin diffusion length, E (=âj/Ï) is the electric field, j is the current density and Ï is the conductivity of Pt. The areal spin density ns transferred into Fe is obtained as \({n}_{\text{s}}={u}_{\text{s}}^{0}\lambda N\) (ref.â18), where N is the density of states at the Fermi level. Using Nâ=â6âÃâ1048âJâ1âmâ3, λâ=â4ânm, ξâ=â0.06, Ï â=â2.0âÃâ106âΩâ1âmâ1, nsâ=â4.2âÃâ1012âμBâcmâ2 is obtained for Iâ=â1âmA. As Fe has a bcc structure (lattice constant aâ=â2.8âà ) with a moment of about 1.0âμB for tFeâ=â1.2ânm at room temperature64, the areal density of the magnetic moment of Fe nFe is determined to be 2.6âÃâ1014âμBâcmâ2. In this case, the filling of the d-band by spin current leads to a change in the magnetic moment of the order of ns/nFeâââ0.16%, which agrees with the ratio between ÎHK and HK, that is, ÎHK/HKâââ2.0âmT/ 1âTâââ0.2%.