Precision spectroscopy on 9Be overcomes limitations from nuclear structure

Historically, advances in precision atomic spectroscopy have progressed alongside the development of the quantum theories of nature to successfully explain even the smallest of contributions to atomic transition frequencies. Meanwhile, precise tests and searches for new physics require that the established theories provide sufficiently accurate predictions for the observed system. Nowadays, in atomic systems, this frequently necessitates the knowledge of nuclear properties. However, these properties are often not known accurately enough from theoretical models describing the nuclear structure and are instead determined experimentally from independent measurements with high sensitivity to nuclear structure. For example, measurements of the Lamb shift in atomic⁶ or muonic hydrogen⁷ and deuterium⁸ are combined with other transitions to independently determine the proton charge radius and the Rydberg constant⁹. However, discrepancies between competing results remain unsolved¹⁰. Likewise, the magnetic dipole–dipole interaction of the nucleus with the bound electrons, which results in the hyperfine splitting (HFS), depends on the magnetic moment of the nucleus and is markedly influenced by the Zemach radius—a measure of electric and magnetic form factors of the nucleus. Here measurements of the 1s-HFS interval in hydrogen-like systems are the most sensitive to the Zemach radius and serve as ideal references to evaluate the nuclear structure effects in other HFS intervals and test the quantum electrodynamics (QED)¹¹. However, for low nuclear charge Z, these measurements exist only for the hydrogen isotopes¹¹ and ³He (refs. ^4,12), whereas for high Z, tests of the HFS lack accurate experimental values of the nuclear magnetic moments^13,14,15.

Recently, the high-precision Penning-trap measurement of the Zeeman and hyperfine splitting of ³He⁺ allowed us to directly measure its Zemach radius and nuclear magnetic moment⁴, simultaneously providing both parameters needed for precise predictions of other HFS intervals¹². Moreover, the accurate value of the magnetic moment of the atom enables absolute magnetometry with hyperpolarized ³He (ref. ¹⁶). However, this requires transferring the measured nuclear magnetic moment from the hydrogen-like system to the neutral system, which involves the theoretical calculation of diamagnetic shielding parameters. In the past, inadequate calculations of these parameters have led to several discrepancies in precision physics^13,17,18,19, including the recent 7σ deviation of the HFS specific difference in ²⁰⁹Bi^82+,80+. In these studies, the required nuclear magnetic moments were obtained from measurements using systems with complex electronic structures and calculations of shielding parameters relying on quantum chemistry codes that frequently provide no, or underestimated, uncertainties¹⁵. By contrast, for systems such as hydrogen-like and neutral ³He, these issues are remedied by the simple electronic structure, which enables diamagnetic shielding calculations using highly accurate non-relativistic quantum electrodynamics methods. Here the diamagnetic shielding parameters are calculated in a perturbative approach, and the estimated uncertainties are more than one order of magnitude better than the experimental value of the ³He nuclear magnetic moment²⁰. However, adjustments to the non-relativistic quantum electrodynamics theory value at the same level as the experimental uncertainty were performed recently²¹, further motivating an experimental verification and benchmark for diamagnetic shielding calculations.

An ideal candidate to both test the diamagnetic shielding calculations and introduce a highly accurate reference for nuclear structure contributions in the hyperfine interaction is ⁹Be. Here the low nuclear charge of Z = 4 permits calculations of the highest available accuracy in the hydrogen-like system, while, simultaneously, the Zeeman and hyperfine splitting can be probed using high-precision spectroscopy in Penning traps for both the lithium-like ⁹Be⁺ and hydrogen-like ⁹Be³⁺ charge states. In this work, we present the first measurements, to our knowledge, on ⁹Be³⁺. Compared with extractions using ⁹Be⁺ (refs. ^5,22), the higher accuracy of theoretical calculations possible in hydrogen-like ⁹Be³⁺ allows for markedly improved determinations of the Zemach radius as well as the magnetic moment of the bare nucleus. Furthermore, we perform a unique comparison between the experimental hyperfine and Zeeman splitting of ⁹Be⁺ and ⁹Be³⁺, which we use to eliminate the nuclear structure-dependent terms. Compared with the measurements of the hyperfine splittings of ²⁰⁹Bi^82+,80+ (ref. ¹³), this allows not only testing the QED theory using the HFS specific difference but also testing calculations of multi-electron diamagnetic shielding parameters at the parts per billion (ppb) level. The latter constitutes the first precision test of the corrections to nuclear magnetic moments across different charge states.

The combined hyperfine and Zeeman interaction in ⁹Be³⁺ is described by the Hamiltonian

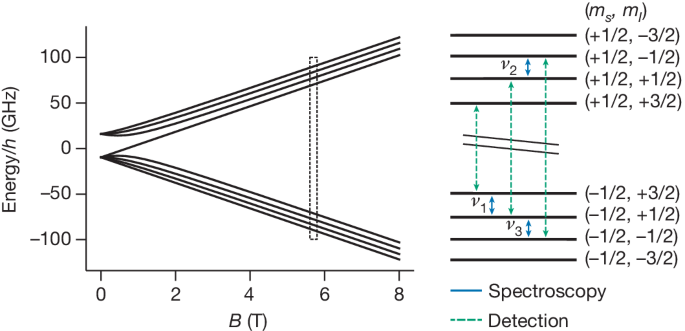
where e is the elementary charge; m_e and m_p are the electron and proton masses, respectively; and ν_HFS is the hyperfine splitting. The external magnetic field B is chosen to define the z-direction as the quantization axis for the spin angular momenta of the electron S and nucleus I. In this formula, the magnetic moments of the bound electron and shielded nucleus are given in units of the Bohr and nuclear magneton using the gyromagnetic ratios (g-factors), g_s and g′_I (the prime indicates the shielding), respectively. Using the spin magnetic quantum numbers of the electron, m_s, and the nucleus, m_I, the level structure of ⁹Be³⁺ is shown in Fig. 1. The 12 magnetic dipole transitions can be split into six low-frequency, 5.7 GHz < ν < 7.2 GHz, nuclear spin transitions with (Δm_s, Δm_I) = (0, 1); four high-frequency, 141 GHz < ν < 181 GHz, electron spin transitions with (Δm_s, Δm_I) = (1, 0); and two high-frequency combined transitions with (Δm_s, Δm_I) = (1, 2). The highest sensitivity for the extraction of g′_I and ν_HFS is reached with the measurement of the two nuclear transitions ν₁ ≈ 6.622 GHz and ν₂ ≈ 6.553 GHz (compare Fig. 1). Our determination of the magnetic field B requires the precise knowledge of the mass \({m}_{{}^{9}{\rm{Be}}^{3+}}\) of the ⁹Be³⁺ ion. As the uncertainty \(\delta {m}_{\genfrac{}{}{0ex}{}{9}{}{\rm{Be}}}/{m}_{\genfrac{}{}{0ex}{}{9}{}{\rm{Be}}}=9\times 1{0}^{-9}\) of the current accepted mass value²³ would limit the extraction of g′_I, the measurement of a third nuclear transition ν₃ ≈ 6.124 GHz was included, allowing us to independently determine the mass.

On the left, the energies of the spin states are shown as a function of the external magnetic field B. The level structure at our magnetic field (dashed box) of around 5.7 T is shown on the right. Spectroscopy is performed on the three nuclear spin transitions (blue) labelled ν₁, ν₂ and ν₃. The three electron spin transitions (green) are used for detecting the nuclear spin state.

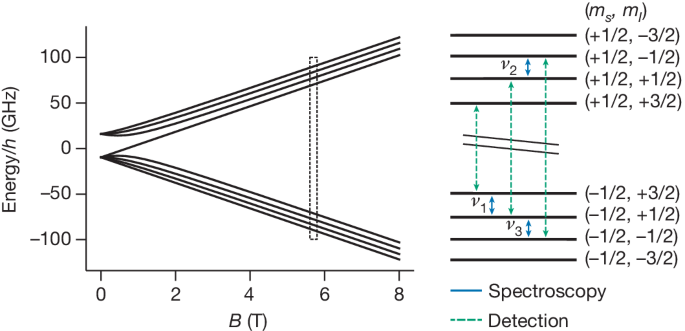
The measurements are performed with a single ion in the Penning-trap setup shown in Fig. 2. The setup is placed in a sealed-off vacuum chamber inside the liquid-helium-cooled bore of a 5.7-T superconducting magnet. Inside this chamber, the vacuum conditions allow for trapping lifetimes exceeding the multiple months needed for the full measurement campaign. The magnetic field confines the ion on a circular orbit with revolution frequency \({\nu }_{c}=(qB)/(2{\rm{\pi }}{m}_{{}^{9}{\rm{Be}}^{3+}})\), where ν_c is the cyclotron frequency and q = 3e is the charge of ⁹Be³⁺. The cylindrical electrodes of the trap are biased by an ultrastable voltage source to create a quadrupolar electrostatic potential, forcing the ion into a harmonic oscillation along the z-axis with frequency ν_z. This further splits the radial motion into two eigenmotions characterized by the modified cyclotron frequency ν₊ and the magnetron frequency ν_−. Here ν_c ≈ ν₊ ≈ 29 MHz ≫ ν_z ≈ 480 kHz ≫ ν_− ≈ 4 kHz. By measuring the three eigenfrequencies and combining them using the so-called invariance theorem, \({\nu }_{{\rm{c}}}^{2}={\nu }_{+}^{2}+{\nu }_{-}^{2}+{\nu }_{z}^{2}\), the cyclotron frequency is reproduced while cancelling certain systematic effects²⁴. A superconducting tank circuit is connected to one of the trap electrodes to provide resistive cooling of the axial mode to the ambient 4.2 K as well as detection of the axial oscillation²⁵ (Fig. 2). Sideband coupling to the axial mode enables thermalization and frequency measurement of the radial modes²⁶.

The Penning trap is built up of gold-plated copper electrodes separated by isolating sapphire spacers (blue). Microwaves for driving the electron spin transitions are irradiated on-axis by a waveguide, and a coil with a few windings connected to coaxial cables is used to drive the low-frequency nuclear transitions in the precision trap. ⁹Be³⁺ ions are produced using laser ablation from a solid beryllium target and subsequent electron impact ionization. a, While alternating the irradiation of the two electron spin detection transitions (indicated by the two background colours) in the analysis trap, axial frequency jumps are observed only for one of them. b, The motional frequencies of ⁹Be³⁺ are measured by dip signals in the Fourier spectrum of the detection signal. a.u., arbitrary units.

For the spectroscopy of the spin transitions, we count spin-state changes of the ion following an excitation with a frequency close to the transition centre. Changes in the spin state are detected by using the continuous Stern–Gerlach effect²⁷. To this end, a ferromagnetic ring electrode introduces a quadratic magnetic field ΔB = B₂z², where B₂ ≈ 282 kT m^−2. This couples the magnetic moment of the ion to its axial motion, slightly altering ν_z depending on the spin state. Changes in the spin state induced by a transition (m_s, m_I) → (m′_s, m′_I) lead to a shift Δν_z proportional to the change of the magnetic moment²⁷. For an electron spin transition, (Δm_s, Δm_I) = (1, 0), the axial frequency jump Δν_z ≈ 10 Hz can be easily detected. By contrast, the change of the magnetic moment of the ion for a nuclear spin transition, (Δm_s, Δm_I) = (0, 1), is greatly reduced, making its detection challenging. For instance, in the case of ν₁, the axial frequency jump is only Δν_z ≈ 6 mHz, which cannot be discerned from the background fluctuations of ν_z. Instead, for the spectroscopy of nuclear transitions (m_s, m_I) → (m_s, m′_I), the two detection transitions (m_s, m_I) → (m′_s, m_I) and (m_s, m′_I) → (m′_s, m′_I) are used, compare Fig. 1. While cycling these two transitions, only one of them produces detectable electron spin-state changes (Fig. 2a), which unambiguously identifies the nuclear spin state.

As the large B₂ required for spin-state detection would limit the experimental precision, we use spatially separated traps for spin-state detection and the precision measurement, called analysis trap (AT) and precision trap (PT) (ref. ²⁸) (Fig. 2). In the PT, the residual magnetic field inhomogeneity is greatly reduced, B_2,PT ≈ 1 T m^−2. A measurement cycle starts by determining the spin state in the AT. Following an adiabatic transport to the PT, an initial cyclotron frequency measurement ν_c,1 determines the expected spin transition frequency. During a second measurement of the cyclotron frequency ν_c,2, the spin transition is driven with a frequency ν_MW, which is randomly offset from the previously calculated value. After a third measurement, ν_c,3, the ion is transported back to the AT to again detect the spin state and determine whether it changed from the previously detected one. We measured ν_c to a precision of one part in a billion with typical averaging times of a few minutes and performed a single measurement cycle in 20 min. For each of the three transitions, a few hundred measurement cycles were performed.

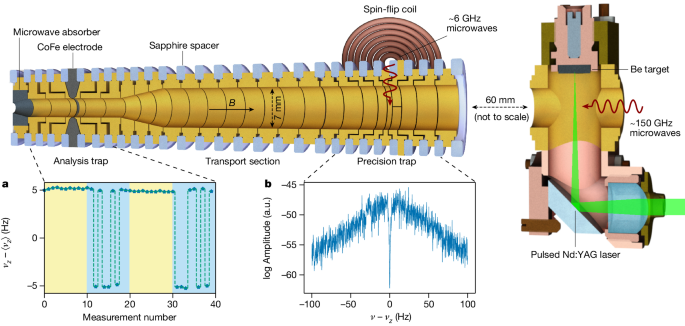
We use maximum likelihood estimation to fit the centre values of ν_MW − ν_i(ν_c∣Γ_e, Γ_I, ν_HFS) from the three recorded resonances shown in Fig. 3 to extract Γ_e, Γ_I and ν_HFS, where

compare equation (1) and Supplementary Information for details. The statistical uncertainties are 17 mHz, 26 mHz and 86 mHz for ν₁, ν₂ and ν₃, respectively. Experimental systematic shifts and uncertainties are because of special relativity effects, electrostatic and magnetostatic field imperfections, induced image charges, the axial frequency determination and the accuracy of the GPS-locked rubidium clock. Moreover, we include a second-order effect arising from the electric quadrupole moment of the nucleus²⁹, slightly shifting the transition frequencies. A discussion of systematic effects and the error budget is provided in the Supplementary Information. The corrected results are Γ_e = −5479.8633435(11)(19) Hz, Γ_I = 2.1354753854(11)(3) × 10^−4 Hz and ν_HFS = − 12796971342.630(50)(15) Hz, where the first number in parentheses is the statistical error and the second is the systematic uncertainty.

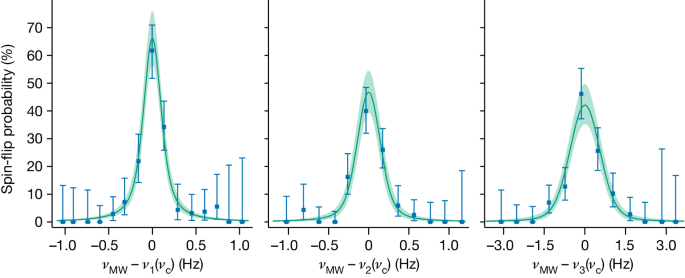
The difference of the probe excitation at frequency ν_MW to the nuclear spin transition frequency ν_i(ν_c) is calculated from the simultaneously measured ν_c. The data points are the binned number of successful spin-flip tries divided by the total number of tries in that bin, and the error bars correspond to the 68% binomial confidences. The line and confidence band (68%) are taken from a Voigt profile fit by maximum likelihood analysis of the unbinned data.

Both the g-factor of the free electron and of the bare nucleus need to be corrected for the influence of the binding potential in the composite system³⁰. Our calculations of the bound-electron g-factor include corrections due to special relativity, QED, nuclear recoil and structure effects (Supplementary Information). We evaluate g_s(⁹Be³⁺) = −2.0017515747, where uncertainties due to uncalculated higher-order QED corrections as well as nuclear corrections are smaller than 1 in the last given digit. The binding corrections to the bare nuclear g-factor g_I are expressed as g′_I = (1 − σ)g_I, where σ is the diamagnetic shielding parameter. The theoretical calculations of diamagnetic shielding parameters include corrections due to nuclear recoil, relativistic effects, one-loop QED and finite nuclear size effects. We evaluate σ(⁹Be³⁺) = 71.15397(14) × 10^−6 (Supplementary Information).

From Γ_I, we calculate the bare nuclear g-factor using the proton-to-electron mass ratio³¹, our values of the bound-electron g-factor g_s(⁹Be³⁺) and the shielding σ(⁹Be³⁺). Our result, g_I = −0.78495442296(42)_exp(11)_theo, improves the accuracy by a factor of 45 compared with the result in ref. ²², the latter being limited by the shielding parameter of ⁹Be⁺. With a fractional uncertainty of 0.6 ppb, our result establishes the nuclear magnetic moment of ⁹Be as the second most precise, to our knowledge, surpassed only by that of the proton³². By comparisons with ⁹Be⁺, we evaluate the shielding factor of the lithium-like system,

This requires the experimental result of Γ_I(⁹Be⁺) from ref. ⁵ and the bound-electron g-factor g_s(⁹Be⁺) = −2.0022621287(24). For the latter, we use the calculations performed in ref. ²² and the updated nuclear recoil correction^33,34. We evaluate σ(⁹Be⁺) = 141.8821(11)_exp(12)_theo × 10^−6, where the second uncertainty is limited by g_s(⁹Be⁺). The theoretical value, σ(⁹Be⁺) = 141.85(3) × 10^−6 (ref. ²²), is in good agreement with our experimental result. To our knowledge, this constitutes the first high-precision test of the calculation of a multi-electron diamagnetic shielding parameter. The shielding calculations for the three-electron systems ⁹Be⁺ and ^6,7Li are performed identically and use explicit values of the leading- and lowest-order recoil terms and an estimate of the relativistic correction^22,33. At the current state of theoretical calculations of the lithium-like shielding parameters, we confirm the leading-order calculation and the estimate of the relativistic correction, solidifying its use for ^6,7Li. In the future, the advanced calculations performed for ³He can be extended to ⁹Be⁺ (ref. ³⁵), and our experimental value of σ(⁹Be⁺) will serve as an ideal benchmark at the ppb precision level.

Calculations of the zero-field hyperfine splitting can be expressed as^36,37,38

where E_F is the non-relativistic value of the hyperfine splitting³⁹, a₀ is the Bohr radius, δ_pt summarizes all corrections with a point-like treatment of the nucleus (Supplementary Information), and all nuclear structure contributions are absorbed in \(-2Z{\widetilde{r}}_{Z}/{a}_{0}\approx 6\times 1{0}^{-4}\) by the effective Zemach radius \({\widetilde{r}}_{Z}\). In comparison, the relative nuclear structure contributions to the electron g-factor g_s and the shielding σ(⁹Be³⁺) are below 10^−10, which highlights the sensitivity of the HFS to effects from the nuclear structure. From our experimental result, ν_HFS(⁹Be³⁺) = −12796.971342630(52) MHz, we calculate the effective Zemach radius \({\widetilde{r}}_{Z}=4.048(2)\,{\rm{fm}}\). This value is consistent with that extracted from ⁹Be⁺, \({\widetilde{r}}_{Z}=4.03(5)\,{\rm{fm}}\) (ref. ³⁶) (value corrected with our more accurate magnetic moment) and improves its accuracy by a factor of 25, which is possible because of the more accurate calculation of δ_pt(⁹Be³⁺) compared with δ_pt(⁹Be⁺).

Following investigations on ²⁰⁹Bi (ref. ¹⁹), we form a specific difference between the hydrogen- and lithium-like systems, Δν_HFS = ν_HFS(⁹Be⁺) − ξν_HFS(⁹Be³⁺) to cancel the large theoretical uncertainties due to nuclear structure with the calculated weighting factor ξ = 0.04881891046 (Supplementary Information). This complements the high-Z case of ²⁰⁹Bi because of the different theoretical approaches used to calculate the lithium-like HFS. For high Z, the large relativistic effects are included directly in the leading order by the use of a relativistic wave function basis set, whereas electron–electron correlations are treated perturbatively⁴⁰. By contrast, for low Z, the electron–electron correlations lead to considerably larger contributions that require using wave functions constructed from an explicitly correlated, non-relativistic basis set, and the relativistic corrections are instead treated perturbatively^41,42. Therefore, in the case of ²⁰⁹Bi, higher-order QED terms are tested by Δν_HFS, whereas in our study at low Z, higher-order electron correlation effects are benchmarked. The experimental result, using ν_HFS(⁹Be⁺) = −625.008837044(12) MHz from ref. ⁴³ is Δν_HFS,exp = −274.638909(12) kHz, where the uncertainty is dominated by ν_HFS(⁹Be⁺). We calculate the theoretical value, Δν_HFS,theo = −271.4(3.6) kHz, which is in good agreement with the experimental value but has a much larger uncertainty. Equivalently, this constitutes a test of ν_HFS(⁹Be⁺) with 6-ppm precision. Similar to calculations for ^6,7Li, estimates of the QED contributions to the lithium-like system limit the accuracy of the theoretical result³⁸.

In Fig. 4, we compare the nuclear structure resolution of several measurements. Our determination of the nuclear structure contribution to the HFS of ⁹Be by ⁹Be³⁺ shows leading experimental resolution and substantial improvements of the theory compared with ⁹Be⁺. This progress now enables testing other HFS systems in ⁹Be with higher accuracy using our precise values of the Zemach radius and the nuclear magnetic moment, as demonstrated by our evaluation of the specific difference. Furthermore, future tests of the theory of the HFS in the helium-like system ⁹Be²⁺ will benefit from our results⁴⁴, and direct measurements of the 2s HFS in ⁹Be³⁺ would provide an opportunity for highly stringent tests of bound-state QED because of the more accurate theory in single-electron systems.

The y-axis is the fraction of the theoretical uncertainty of the point-nucleus calculations to the nuclear structure contribution. The x-axis is the fraction of experimental uncertainty to the nuclear structure contribution. The dashed diagonal line indicates equal experimental and theoretical resolution. For H, D and T, HFS calculations from ref. ³⁸ were used, which, as opposed to our value for ν_HFS(⁹Be³⁺), do not include contributions and uncertainties from hadronic and muonic vacuum polarization as well as certain nuclear recoil terms. For comparison, the blue dashed line and point indicate the reduced theoretical uncertainty of ν_HFS(⁹Be³⁺) using the same calculations that do not include the aforementioned contributions. For details, see Supplementary Table 7.

Finally, we calculate the atomic mass of ⁹Be from Γ_e, using the electron mass³¹, the required binding energies^45,46,47 and our theoretical value of g_s(⁹Be³⁺). The result, \({m}_{{}^{9}{\rm{Be}}}=9.0121830344(35)\,{\rm{u}}\), is in perfect agreement with the accepted value²³ and improves the uncertainty by a factor of 20.

The results are summarized in Table 1. In conclusion, our precision measurement of the bare nuclear magnetic moment and the effective Zemach radius of ⁹Be with hydrogen-like ⁹Be³⁺ enables tests of QED methods accessible only with accurate knowledge of these properties. At present, by comparison with measurements on ⁹Be⁺, we provide the, to our knowledge, first high-precision test of multi-electron diamagnetic shielding calculations and a 6-ppm test of the QED calculations of the lithium-like 2s HFS. High-precision Penning-trap measurements of hyperfine and Zeeman splittings, which we previously demonstrated for ³He⁺, are now possible for a multitude of other hydrogen-like or lithium-like ions, enabling the suppression of nuclear effects, mandatory for further applications such as spectroscopic searches for physics beyond the standard model⁴⁸. Furthermore, measurements on ^6,7Li²⁺ would enable direct comparisons with the recent measurements on the helium-like charge states^21,37,49.