Herring spawned poleward following fishery-induced collective memory loss

Catch data

The catch data used in this study, while not comprehensive in representing the entire distribution of NSS herring, serve as a crucial source for understanding spatiotemporal dynamics. Our analysis integrates catch data from Norway, Iceland and the Faroe Islands, accounting for approximately 80% of total landings spanning the years 1995 to 2023. These data are reported annually to the International Council for the Exploration of the Seas (ICES) quarterly and organized within ICES rectangles (0.5° latitude and 1° longitude)⁵². Our analyses on the changes in distribution and the defined seasonal migration culture within the population were based on monthly aggregated data per ICES rectangle prepared by each nation for the study.

Furthermore, to demonstrate the long-term stability in spawning at the west coast of Norway followed by an abrupt poleward shift, we calculated the COG in the Norwegian fishery over 14–29 February, representing fish that have arrived at their designated spawning location⁵³. These data were restricted to the period 2000 onwards, when the Norwegian catch data were available at the level of individual landings from the Norwegian Directorate of Fisheries (Fangstdata (seddel) koblet med fartøydata (åpne data), Fiskeridirektoratet; https://www.fiskeridir.no/statistikk-tall-og-analyse/data-og-statistikk-om-yrkesfiske/apne-data-fangstdata-seddel-koblet-med-fartoydata). The COG was calculated as the arithmetic mean of midpoint positions in statistical rectangles (system of the Norwegian directorate of fisheries, mostly 0.5° latitude and 1° longitude) weighted by the corresponding total catch within the rectangles and the specified date interval. Note that we describe the temporal dynamics in COG only on the latitudinal scale.

Population dynamics data

Numerical dominance of recruiting cohorts in the population as well as longer term trends in spawner biomass, catch, fishing mortalities and exploitation patterns, were described based on data available from the assessment reported by ICES⁵², covering the period 1988–2023.

Note that the assessment of NSS herring has been considerably revised over this period following changes in input data and model framework. Thus, retrospective patterns in the perception of trends in spawner biomass were demonstrated by adding data from the 1999 and 2009 ICES NSS herring assessments^54,55.

We defined numerical dominance of a cohort as when the proportion of fish aged 4 years among fish aged 4 years and older exceeds 0.5. Here the 2016 cohort stands out as the most numerically dominant one over the period 1988–2023. Our main hypothesis was that this numerical dominance led the cohort to take its own decisions, and that the older fish, following high fishing pressure and plummeting, abundance adopted the newly established migration culture. To illustrate how the 2016 cohort left its Barents Sea nurseries and recruited to the spawning population over the period 2017–2023, we presented the proportion in abundance estimates from specific acoustic trawl surveys covering the full feeding distributions in the Norwegian Sea in spring (IESNS survey) and summer (IESSNS survey) as well as the spawning season (Spawning survey) based on data from the recent assessment report⁵².

Moreover, given the expected importance of mixing processes between recruits and elders during feeding in the Norwegian Sea, spatial variation in abundance was detailed as 1 nmi nautical area back-scattering coefficient (NASC)⁵⁶ values along the defined transects during the IESNS and IESSNS surveys. Further details from these surveys and the methodology used is available in full survey reports attached in the annual ICES herring assessments in 2017–2023^{52,57,58,59,60,61,62}.

One parameter linked to population dynamics of specific relevance to migration potential is body growth^20,26. Fluctuations in growth during 1988–2023 were analysed using IMRs biological data on total body lengths (L) in cm from fully recruited 7-year-old individuals sampled during quarter 1 (n = 17,609) collected in the commercial fishery and research vessel trawl hauls.

Another parameter having significant effect on spawning migration specifically is the amount of energy reserves available in this non-feeding period^3,26. Thus, the corresponding trends in body condition were analysed with a combination of L and total weight (W) in g of individual fish, using Fulton’s condition factor (CF =W/L³ × 100)⁶³, to characterize the energetic status. Here we included all data from maturing fish (L ≥ 27 cm⁶⁴) in January (n = 36,501), which should represent the initial condition of the population at the onset of spawning migration.

Spatiotemporal cohort data

An important assumption of our study is that the effects of numerical domination for learning processes and transfer of migration culture occur at the school level. To explore the spatiotemporal development in numerical dominance of the 2016 cohort in schools linked to the abrupt poleward shift in spawning, we analysed a vast material of biological samples (n = 3,226) from single-trawl hauls and purse seine sets over the period 2017–2024. These were from Norwegian, Icelandic and Faroese fisheries as well as relevant surveys, including the international ecosystems surveys in the Norwegian Sea in spring (IESNS) and summer (IESSNS) and the spawning survey in February. We filtered the samples containing randomly aged individuals from the 2016 cohort and older fish (total n = 91,878) assumed to represent the cohort structure within a school. The spatiotemporal dynamics of the 2016 cohort at the school level were then demonstrated by mapping the proportion of this cohort in all samples quarterly over annual migration cycles, starting during the feeding season in quarter 2 and ending during the spawning season quarter 1.

A similar analysis was conducted for the available samples (n = 1,748) and aged fish (n = 61,616) of the 2002 cohort and older fish during the period that the 2002 cohort recruited to the spawning population (2003–2010). This comparative analysis was relevant as the 2002 cohort wintered at same latitudes as the 2016 cohort but still migrated all the way to spawn off the Norwegian west coast. To illustrate how body growth influenced the progress of southward spawning of the 2002 cohort, we first described how it gradually became numerically dominant in acoustic trawl surveys conducted during late autumn in the northern wintering areas over the years 2004–2006 according to data from the 2007 ICES assessment⁶⁵. Second, we showed how these dynamics were related to growth based on IMR biological data on development in body lengths of this cohort during the wintering situation in quarter 4 over the years 2004–2006 (n = 1,781). Third, we demonstrated how the proportion of the cohort progressed with the distance of the spawning migrations on a latitudinal range and over the years 2005–2007 based on aged individuals from quarter 1 (n = 8,135). Finally, we addressed how these dynamics corresponded with dynamics in body lengths of the 2002 cohort (n = 2,338).

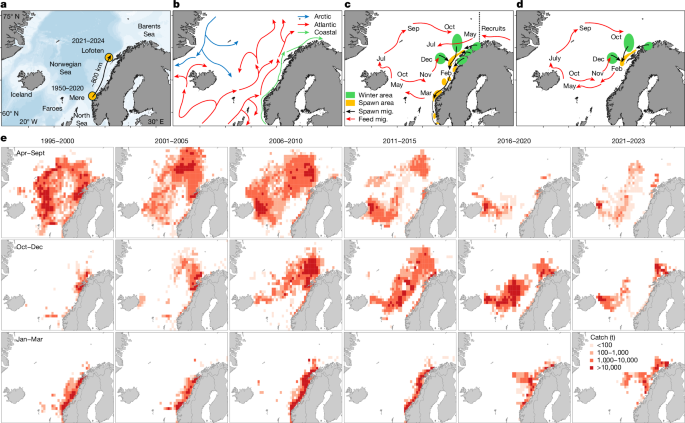
While both data on fisheries and fraction of the 2016 cohort in schools served as evidence for an abrupt poleward shift in spawning of NSS herring, the main quantification of this process was derived from the Spawning surveys during 2018–2024. All details from these surveys and the methodology used is available in full survey reports attached in the annual ICES NSS herring assessments in 2018–2023^{52,58,59,60,61,62}, and in the 2024 IMR survey report⁶⁶. Note that, in these years, the execution of the acoustic trawl surveys was directly comparable, running northwards against the migration direction covering the full distribution within the confined spawning areas over around 10 days and the same dates (14–25 February) using either three (2018–2020) or two vessels (2021–2024). The survey transects were specifically designed to maintain a high degree of coverage, with trawling regularly on the acoustic registrations for biological sampling and ageing of ~50 specimens per haul. The saware (StoX⁶⁷) and statistical approach was used to estimate the cohort abundance within prespecified strata for all surveys. The poleward shift in distribution linked to the spatiotemporal development in numerical dominance of the 2016 cohort was explored by comparing the acoustic abundance relative to the aggregated abundance of older and younger fish as pie charts at strata midpoints in maps. Here the size of pies was weighted to the highest acoustic abundance estimate at any strata within each survey year.

Tag-recapture data

In the present study, we also demonstrate the migration behaviour of individually tagged herring to support the observed dynamics at the population and school level. The tagging program on NSS herring using RFID technology was initiated by IMR in 2016 for assessment purposes and migration studies. All data relevant to the tagging program are open to the public through APIs⁶⁸. 

During 2016–2023, herring was tagged on annual basis over a period of 3 weeks in the wintering areas in fjords of northern Norway during November–January. PIT tags, type ISO FDX-B 134.3 kHz, 3.85 × 23 mm biocompatible glass tags are used in the experiments. Herring were tagged at random ages 2–20 years, 20–24 cm body lengths and approximately 50% of each sex. The total number tagged on an annual basis (total sample size in experiment) was estimated to be within a range that gives adequate uncertainty levels when data are used as input to age-based assessments of population size.

IMR rents a commercial purse sein vessel for the tagging surveys. Here herring are captured on daily basis with purse seine early in the morning and pumped gently onboard to the refrigerated sea water storage tanks though pipes with sea water. These tanks are normally used to store the catch cold until landed at a factory, but here they are specially equipped with small keeping nets for the purpose of holding the live herring until tagging. From these tanks, the individual fish is dip-netted and tags are injected into the abdomen. All tagged fish are transferred to a smaller storage tank and released into the sea in schools of 200 individuals. Regarding animal welfare, the tagging experiments are approved by the Norwegian Food Safety Authority (FOTS), which handles all applications to use animals in scientific experiments. During the tagging process, a PC-reader system with RFID antenna continuously records the unique tag IDs together with the body length and other details relevant for the experiment, which frequently are synchronized with an IMR database over internet. Moreover, biological samples with age and length measurements forms the basis for forward age length keys describing age probabilities on the basis of size⁶⁹, which is used to estimate numbers released by each cohort.

The PIT-tagged herring are later recaptured at Norwegian and Icelandic factories producing landings from the commercial fishery for human consumption. Here several factories are equipped with monitoring systems including RFID antennas specially designed for pipes (round antennas) or conveyor-belt systems (flat antennas) detecting tagged fish during the production process. These antennas are connected to PC-reader systems that communicate directly with the IMR database providing updated information of recaptures in real time.

Moreover, all relevant data from the catches scanned for PIT-tags, including vessel info, catch quantum, catch position (ICES rectangle), catch date and production time, are uploaded to the database at a later stage. Finally, allocations between recaptures and catches are based on the combined info from time of recapture and the specific catch produced at that same time. In this study, we present information on the distribution and biomass of all the catches scanned for tags, as well as the distribution of recaptures from all the experiments 2016–2023.

Bioenergetic migration model

NSS herring do not feed during spawning migration, they rely on stored reserves²⁶. The energy used during the spawning migration was modelled using a simple migration model coupled with a respiration model. In the migration model, the fish follow a predetermined route defined from the observed distribution of 1 nmi NASCs during the acoustic trawl survey in 2018⁵⁸. For each latitude increment of 0.5°, the COG was calculated, resulting in 14 locations, connected by 13 transects and with a total swimming route length of 927 km.

The southward spawning migration of NSS herring is constrained by the external forces of the coastal currents. In the migration model, the interannual variations in coastal currents were included in terms mean velocities (u and v components) along each of the 13 transects of the migration route in 2020–2023. The velocities were obtained from the ORAS5 reanalysis produced by the European Centre for Medium-Range Weather Forecasts (ECMWF) provided at 0.25° × 0.25° grids averaged over January–February⁷⁰. The ocean currents on the Norwegian shelf and continental slope are largely wind driven⁷¹. The Norwegian Atlantic Slope Current has little vertical shear over the upper 300 m (ref. ⁷²), where the herring migrate⁵⁸. We therefore assume that the surface currents are representative of the velocities that herring were facing during upstream spawning migration.

Simulations were performed for observed L and W for the 2016 cohort of herring in 2020 (27.5 cm, 168 g), 2021 (29 cm, 210 g), 2022 (30 cm, 242 g) and 2023 (31.5 cm, 284 g) measured at onset of spawning migration in January. The condition was described using Fulton’s condition factor. The dry weight (DW) was then calculated on the basis of a water content of 68%, and the DW was partitioned into 15% gonads and 85% somatic tissues with equal parts of fat and solids, typical for this time of year^26,73. The total initial energy was then calculated based on energy of gonads (25 kJ g⁻¹ DW) derived from the 68% water content and known wet weight energy (8 kJ g⁻¹ WW⁷³), and the energy of somatic tissues using the known energy of fat (39.75 kJ g⁻¹ DW)⁷⁴ and solids (20.92 kJ g⁻¹ DW)⁷⁴.

During migration, the fish was set to swim with a net swimming speed of 1 body length per second, consistent with the observed migration speed of NSS herring between wintering and spawning areas⁵³ as well as cruising speed in tank experiments⁷⁵. Moreover, at each transect, the swimming speed was increased proportionally to the counter current speed. Specifically, this was done by first calculating the swimming time (t₀) of a transect assuming no currents. The fish was then advected for this amount of time, and the actual distance under the influence of currents calculated. Lastly, the actual swimming speed (t₁) was increased to account for the extra swimming distance imposed by advection (t₁ = t₀).

Respiration loss (\({R}_{{{\rm{O}}}_{2}}\), grams O₂ per g per day) was calculated using a classical Hewett and Johnson model⁷⁶:

where f(W) is the weight dependence on respiration, f(T) is the impact of temperature on resting metabolism, and f(SS,L) is the impact of active metabolism (that is, swimming activity). The weight dependence was represented by an allometric scaling function as:

where W is the fish weight and α and β are the intercept (0.0033 g O₂ per g per day) and slope (−0.227), respectively. The temperature dependence was calculated as an exponential function as:

where T is the water temperature, set to 8 °C according to results of the present study, and θ is a constant (0.0548 °C⁻¹) describing the impact of temperature on the resting metabolism. The impact of swimming activity was calculated as an exponential function as:

where SS is the relative swimming speed, Lis the length of the fish and T_o is a constant (0.03 °C⁻¹) describing the impact of swimming on the active metabolism. Finally, the specific respiration loss was converted to specific energy loss using an oxy-calorific coefficient of 15.062 J g⁻¹.

During the simulations, energy consumption for swimming was extracted from the somatic tissue fat pool. If the fat pool was depleted, the fish would shift to using solids. Moreover, energy was transformed from somatic tissues into gonads at a rate of 0.01 d⁻¹ until they constituted 20% of total DW, a typical level for pre-spawners in February²⁶.

The costs in terms of loss in W and total energy were predicted along the full migration route of 13 transects, although this evidently would not be feasible to survive for sequential spawning events. To predict the actual migration potential the condition factor after spawning (CF*) was also calculated between each transect by subtracting the gonad weight from the total weight. CF* was then used to assess how far the 2016 cohort would be able to swim in the years 2020–2023 before having to spawn assuming a lower threshold of CF* = 0.60. This threshold was set according to the observed 10th percentile of CF* among spent herring ≥27 cm analysed by IMR over the period 1935–2023 (n = 26,452). We assume that surpassing this threshold would increase the risk of mortality.

Zooplankton biomass and temperature data

To examine whether the recent abrupt poleward shift in NSS herring spawning could be linked to changes in the biotic and abiotic environment, we analysed IMRs 30-year long time series (1995–2024) of zooplankton data from WP2 net hauls at three cross-sections (Svinøy, Gimsøy and Fugløy) and temperature data from conductivity, temperature and depth (CTD) casts at three monitoring stations (Bud, Eggum, Ingøy) overlapping with the period from onset of spawning migration 15 January⁵³ until the time when most offspring have reached metamorphosis 30 June³.

IMR temperature data from CTD casts at monitoring stations are publicly available (https://www.imr.no/forskning/forskningsdata/stasjoner/view/initdownload), with records at standard depths. We used data from depths of 1, 5, 10, 20, 30, 50, 75, 100, 125, 150 and 200 m in our analyses.

The zooplankton data were extracted from IMRs local data. They were collected with WP2 nets using mesh sizes 180 μm, according to the standard procedure for the surveys. The net was hauled vertically from 200 m to the surface or from the bottom whenever bottom depth was less than 200 m. The exception was stations at the Fugløy cross section, where standard hauls start at 100 m depth. All of the samples were sieved into the size fractions 180–1,000 µm, 1,000–2,000 µm and >2,000 µm, dried and weighed and data presented as g DW per m². In our analyses, we included only the data from the smallest size fraction of zooplankton (180–1,000 µm) as indices for the prey availability for larvae.

For each time series, we fitted separate GAM models to the data. Zooplankton biomass were log-transformed before analysing the effects of sampling year and day of year:

For temperature, we added the effect of sampling depth to the model:

We allowed the number of knots k for the sampling year to be as high as possible without overfitting interannual fluctuations and, at the same time, we restricted k for smoothers of day of year and depth where data were expected to follow a clear trend over season.

As we wanted to look at trends in ambient temperatures, we ran two different models for temperature split into depth intervals and periods overlapping with adult spawning migration and incubated eggs on one side (depth = 50–200 m, day of year = 15–90)^40,53,58 and herring larvae from first feeding through metamorphosis on the other (depth = 1–50 m, day of year = 90–180)^3,77,78.

We inspected the GAM-model diagnostics and QQ-plots showed some minor tail issues, especially for ambient temperature of larvae in cases in which there were some high outliers. All models were still considered to be acceptable given that R² values were high (deviations explained were at same level) and all smoothers including the intercepts were significant.

Finally, based on the accepted GAM models, the zooplankton and temperature (T, °C) responses of sampling year, day of year and depth (only for T) were predicted at set values of covariates: year was set to 2021 to represent the poleward shift of spawning, 1 March and 150 m was used as typical time and depth of spawning⁴⁰, 1 April was considered to be the first feeding date of larvae⁷⁷, whereas 1 May and 25 m represented the time and depth of early growth larvae⁷⁸.

Oceanographic currents in study area

In our introduction, we give a schematic overview over main oceanographic currents in our study area (adopted from a previous study⁵¹), to demonstrate the dynamic environment herring is experiencing during it season migrations between wintering, spawning and feeding habitats.

Ethics oversight

We have included data from PIT-tagging experiments on herring. These kinds of experiment fall within the same category as laboratory experiments with animals. All our experiments have been approved by the Norwegian Food Safety Authority (https://www.mattilsynet.no/en). The survey methodologies used for the present study, including the sampling of herring and zooplankton, follow recommendations in protocols from the International Council for the Exploration of the Sea⁷⁹.

Reporting summary

Further information on research design is available in the Nature Portfolio Reporting Summary linked to this article.