Direct experimental constraints on the spatial extent of a neutrino wavepacket

Quantum mechanics is based on the concept that the microscopic scale of our Universe contains inherent uncertainties in position and momentum that are fundamentally connected as σ_xσ_p ≥ ħ/2 (ref. ⁷). Using this theoretical description, the precise knowledge of the momentum width of a particle σ_p implies a corresponding uncertainty in the extent of its spatial wavefunction σ_x. When the object loses its ability to maintain coherence through interactions with the complex environmental bath, its position is localized. This localization of quantum objects heavily depends on the specific environment they are subjected to⁸. Recent efforts have primarily focused on constructing increasingly large coherent quantum systems^9,10 and other highly delocalized objects to push measurement and other applications to—and beyond—the standard quantum limit^11,12. Such experiments are at the frontiers of quantum science and engineering and aim, in part, to probe the interface between classical and quantum mechanics.

Here, by contrast, we present a new measurement concept: using highly localized unstable systems embedded in a complex solid-state material at a low temperature as a precision laboratory to probe the quantum properties of subatomic particles. We show that this concept is particularly powerful for investigating the properties of systems that are otherwise not directly accessible because of weak couplings in the Standard Model of particle physics, particularly the neutrino (ν).

Neutrinos are light, neutral leptons, and the only particles in the Standard Model that have an intrinsic chirality, in that they only interact through left-handed currents of the weak interaction through three flavour eigenstates, namely, ν_e, ν_μ and ν_τ. Neutrino oscillation experiments over the last 30 years^13,14 have also indicated that these flavour eigenstates include at least two non-zero mass eigenstates. This observation of non-zero neutrino masses was awarded the Nobel Prize in Physics (2015), but it is still not known how the Standard Model should be extended to incorporate massive neutrinos¹⁵. The flavour oscillation effect for neutrinos arises from the fact that the mass eigenstates and weak-interaction eigenstates are not equal to each other, and because of their small interaction probabilities with the weak and gravitational forces, they are able to maintain coherence for long distances¹⁶. Thus, even for a neutrino created with a definite flavour—the electron neutrino (ν_e) in weak nuclear decay, for example—a coherent superposition of states exists in the neutrino wavepacket that affects the probability for observing a particular flavour as a function of time or distance from the source. As neutrinos propagate in space, their mass eigenstates separate owing to their different velocities, and eventually no longer overlap resulting in the decoherence of the group wavepacket^17,18,19,20.

As neutrinos rarely interact with matter, they are notoriously difficult to detect. For example, a ν_e with 1 MeV kinetic energy has an interaction cross-section of roughly 10⁻⁴² cm² (ref. ²¹). This fact also makes neutrinos attractive systems to study the fundamental properties of quantum mechanics and exotic new physics, because they are able to maintain coherence for long distances. Historically, observing these so-called ‘ghost particles’ from various sources requires large volume detectors with limited detection precision that run for several years²². These detectors rely on neutral-current and charged-current interactions with an atomic nucleus or electron in these large volumes²¹. The final-state particles are used to reconstruct the incoming neutrino energy and interaction point, along with the neutrino flavour for charged-current interactions. A deficit or excess flux of any flavour in the detector, compared with the flavour ratios at the source, is used to determine the oscillation probabilities as a function of energy and distance. Global data from neutrino oscillation experiments have been used to determine the mass splittings and mixing parameters for the three Standard Model neutrinos¹⁵. Moreover, data from the Daya Bay^23,24, RENO^25,26 and KamLAND²⁷ reactor neutrino experiments currently provide loose indirect constraints on the spatial extent of \({\overline{\nu }}_{{\rm{e}}}\) wavepackets from β⁻ decay sources^5,6; however, ν_e wavepackets from electron-capture (EC) sources have never been studied.

To extract physics from the reactor oscillation experiments above, interpretation in the lepton mixing framework of the Standard Model is required¹⁵. Neutrino mixing parameters, matter effects and combining detector systematic uncertainties make extracting limits on σ_ν,x a complex and difficult task. The current limits on wavepackets from reactor \({\overline{\nu }}_{{\rm{e}}}\) using this approach are 2.1 × 10⁻¹³ m ≤ σ_ν,x ≤ 2 m (refs. ^5,6). If the spatial widths of the \({\overline{\nu }}_{{\rm{e}}}\) and ν_e wavepackets are indeed near the low end of these limits, it has a considerable impact on the neutrino physics landscape, including alleviating tension in models for sterile neutrinos with electronvolt-scale masses through model-dependent fits to the available data^28,29 and impacting the physics goals of JUNO³⁰.

Given the current pervading anomalies in the neutrino physics landscape³¹, there is a need for new techniques that can provide model-independent access to the neutrino. Clever new experimental concepts have been presented to address this need using efficient, small-scale setups^32,33,34 that are less model dependent and not limited by low neutrino interaction cross-sections. These experiments aim to precisely measure the low-energy recoiling atoms in nuclear EC decay³⁵ to access information of the neutrino directly through its entanglement in the final state of radioactive decay. Of these new concepts, the beryllium EC in superconducting tunnel junctions (BeEST) experiment³² is currently the only one to practically use the concept of direct EC daughter recoil detection for neutrino physics³⁶ and astrophysics³⁷. Here we report, to our knowledge, the first direct limit on the spatial width of a neutrino wavepacket from the energy width of the recoiling nucleus in EC decay, σ_N,E, through their mutual entanglement.

Nuclear EC decay is a radioactive decay mode that results from the capture of an orbital electron by a proton in the nuclear volume³⁵. At the fundamental level, this weak-interaction process transmutes an up quark to a down quark through the exchange of a W boson in a constituent nucleon and conserves the lepton number through the emission of ν_e. In the experimental context of the work presented here, the observable final state only contains two products: the recoiling heavy daughter system and ν_e. This simple two-body system that we observe is entangled at its creation, and thus, a precision measurement of the daughter recoil provides direct access to information on the neutrino.

The magnitude of σ_ν,x is expected to depend on environmental factors, the type of decay and the scale of localizing interactions. Predictions based on localization through atomic interactions lead to an estimate of σ_ν,x ≈ 200 nm for \({\overline{\nu }}_{{\rm{e}}}\) from reactor β⁻ decay sources and σ_ν,x ≈ 1–10 μm for ν_e from a ⁵¹Cr EC source^1,3. Other theoretical approaches that assume localization through degrees of freedom in the nucleus for β⁻ decay \({\overline{\nu }}_{{\rm{e}}}\) and degrees of freedom in the atom for EC ν_e lead to σ_ν,x ≈ 10–400 pm for reactor β⁻ decay sources and σ_ν,x ≈ 3 nm for a beryllium-7 (⁷Be) EC source^2,4. As these predictions vary by several orders of magnitude, a precise-enough wavepacket measurement from any nuclear-decay-based ν source could potentially distinguish between these competing theories on the scale of quantum localization in weak nuclear decays.

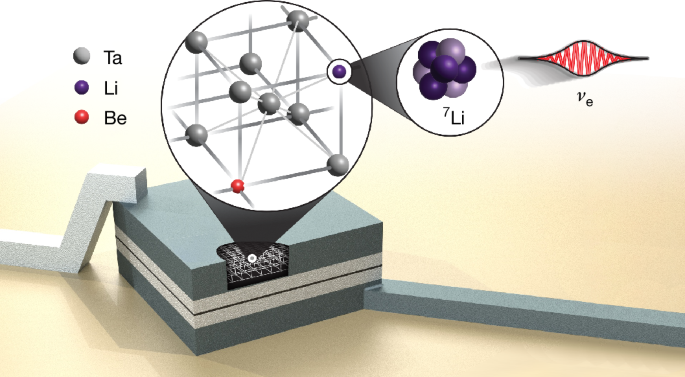
With seven nucleons and four electrons, the neutron-deficient ⁷Be nucleus is the simplest pure EC decaying system in the nuclear landscape. To measure the ⁷Be EC decay spectrum to high precision, we directly embedded the ⁷Be into the top tantalum film of a single superconducting tunnel junction (STJ) that was used as a high-energy-resolution sensor³⁸. The STJ pixel used in this analysis was part of a 36-pixel array in which each sensor had a surface area of 208 × 208 μm² and consisted of the following five layers (from top to bottom): Ta (165 nm)—Al (50 nm)—Al₂O₃ (1 nm)—Al (50 nm)—Ta (265 nm) (Fig. 1). This device was fabricated by photolithography at STAR Cryoelectronics³⁹. STJs exploit the small energy gap in superconducting Ta (Δ_Ta ≈ 0.7 meV) to provide an intrinsic device energy resolution of 1–2 eV in the energy region of interest (approximately 100 eV).

Schematic of the STJ sensor showing the five layers described in the text. The ⁷Be radioactive source is sparsely implanted into the Ta lattice of the absorber layer. The zoomed-in image (top right) shows the final state after EC decay including ⁷Li recoil and ν_e.

Radioactive ⁷Be⁺ ions were directly implanted into the STJ pixel through apertures in a Si collimator that was situated approximately 100 μm above the chip. The ⁷Be⁺-ion beam was produced and delivered to the implantation chamber endstation at the TRIUMF-ISAC facility⁴⁰ at an energy of 30 keV. The isotopically pure (almost 90%) ⁷Be⁺ beam was produced using the isotope separation on-line technique⁴¹ using spallation reactions from a 10 μA, 480 MeV proton beam incident on a stack of thin uranium carbide targets. Once released from the target through diffusion, the created beryllium atoms were selectively ionized using the ion guide laser ion source⁴² operated in suppression mode⁴³. The resulting beam of ⁷Be⁺ ions was implanted at a rate of 6.1 × 10⁶ s⁻¹ for a period of 25 h to generate an initial per-pixel activity in the STJs of roughly 50 Bq. After implantation, the chip was cleaned and processed at TRIUMF to remove loose and surface-deposited activity and subsequently shipped to Lawrence Livermore National Laboratory (LLNL).

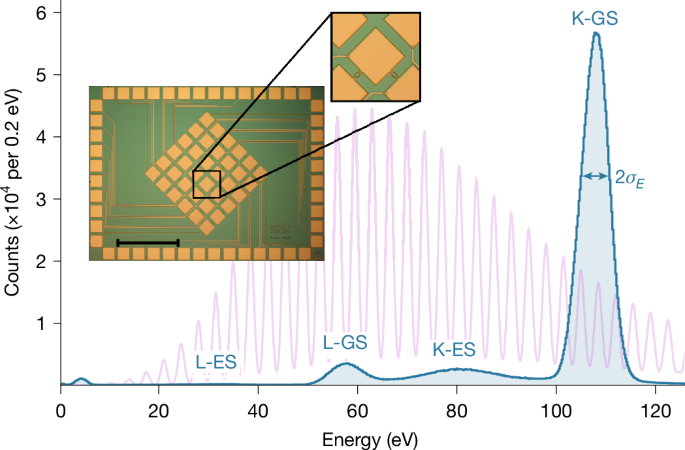
The final-state energy spectrum from the decay of ⁷Be was measured at LLNL with the STJ detector at a temperature of approximately 0.1 K in a two-stage adiabatic demagnetization refrigerator with liquid-nitrogen and liquid-helium precooling. Signal traces were read out continuously at a rate of 1.25 MSa s^–1 at a 16-bit resolution using a PXIe-6356 analogue-to-digital converter. For the real-time in situ monitoring of the response and energy calibration, the STJs were simultaneously exposed to 3.49865(15) eV photons from a pulsed, 355 nm frequency-tripled Nd:YVO₄ laser triggered at a rate of 100 Hz that was fed into the cold stage of the adiabatic demagnetization refrigerator through a fibre to illuminate the pixels. The laser intensity was adjusted such that multiphoton absorption provided a comb of peaks in the energy range from 20 eV to 120 eV. The energy resolution of the laser peaks was 1.85(1) eV at 108.5 eV with residuals of approximately 0.02 eV in the region of interest^44,45. Selected data (Fig. 2) were acquired for approximately 20 h on 6 November 2022, which are a small subset of the full BeEST Phase-III dataset.

The measured ⁷Li recoil spectrum with the four peaks described in the text for 20 h of data from the single STJ pixel shown in the inset. The L-ES peak is barely visible because of its weak population probability. The measured uncertainty of the K-GS peak is shown, and is conservatively extracted as the upper limit on the inherent energy width of the recoil, σ_N,E ≤ 2.9 eV, through the procedure described in the text. The comb of the peaks from the calibration laser spectrum (violet) is also shown for comparison. The small bump in the spectrum at 4 eV results from a non-prompt, decay-induced process, the source of which is still under investigation. Scale bar, 1 mm.

⁷Be decays to lithium-7 (⁷Li) and a ν_e with a half-life of T_1/2 = 53.22(6) days⁴⁶ and a total decay energy of Q_EC = 861.963(23) keV (ref. ⁴⁷). In the EC process, the electron can be captured either from the 1s shell (K capture) or the 2s shell (L capture) of Be, with a measured L/K ratio of 0.070(7) (ref. ³⁷). For K capture, the binding energy of the 1s hole is subsequently liberated by the emission of an Auger electron⁴⁸. A small branch of 10.44(4)% results in the population of a short-lived excited nuclear state in ⁷Li (T_1/2 = 72.8(20) fs) that de-excites by the emission of a 477.603(2) keV γ-ray⁴⁶. As the nuclear decay and subsequent atomic relaxation occur on short timescales, our measurement method results in a low-energy spectrum with four peaks: two for K capture and two for L capture into the ground state (GS) and excited state (ES) of ⁷Li, all of which are well resolved. The two recoil peaks from the ES decay of ⁷Li are Doppler broadened from γ decay in flight^37,38.

The final ⁷Be decay spectrum (Fig. 2) includes events from a single pixel that were not in coincidence with the laser logic pulse or simultaneous signals in other pixels. The latter of these two criteria was imposed to remove the large, low-energy background from 478 keV γ-ray Compton scattering interactions in the 0.5 mm Si substrate below the STJ array, which generated signals in many pixels simultaneously. The resulting EC recoil spectrum is dominated by the four expected peaks arising from the two atomic and two nuclear processes described above. A sophisticated analysis of the spectrum, which includes higher-order processes such as atomic shake-up and shake-off effects, is ongoing for the beyond-Standard Model physics search program of the BeEST experiment, but is not required here.

Only the K-GS peak is used in this analysis because it provides the highest statistical significance for a measurement of the recoil energy width with the smallest uncertainty and does not have Doppler broadening as the ES peaks do. The width of the K-GS recoil peak (Fig. 2) is broadened beyond the intrinsic resolution of the sensors based on the resolution of the laser peaks. Some of this width is because of structural and chemical variations of the Li 1s binding energy at different sites in the Ta lattice, which contribute \({\mathcal{O}}\)(1 eV) to the broadening⁴⁹. However, owing to their complexity and the fact that these effects are not yet quantified in detail for the BeEST energy spectrum, we conservatively attributed all of the measured broadening in the K-GS peak to quantum uncertainty. This also ignores the negligible broadening caused by decays at the edges of the pixel⁵⁰. A future analysis that accounts for known causes of broadening will improve the limits presented here.

The K-GS peak was fit in the region from 101 eV to 115 eV (Fig. 2) with a single Voigt function to extract the energy uncertainty width σ_N,E ≤ 2.9 eV at the 95% confidence level (CL) using the profile likelihood method^51,52,53. We then converted the full energy width to a momentum width of the ⁷Li recoil. The uncertainty was propagated using \({\sigma }_{p}=\sqrt{m/2E}\,{\sigma }_{E}\), where m is the atomic mass of ⁷Li and E is energy of the K-GS peak centroid, resulting in σ_Li,p ≤ 16 keV c^–1. Contributions due to the uncertainty in measurements of m are negligible⁵⁴. Finally, using the Heisenberg uncertainty relation, we extracted a lower limit on the spatial width on the nuclear recoil of σ_N,x ≥ 6.2 pm. These results are based on our measurement of the K-GS peak energy width under the experimental conditions reported here and have no underlying assumptions about the scale of localization. Differences in the expected broadening owing to more realistic non-Gaussian wavepackets are negligible compared with other causes of variations in the broadening mentioned previously².

To extract a limit on the neutrino spatial width σ_ν,x, two approaches were used: one based on the conservation of energy¹ and the other based on the conservation of momentum². In the first method, the neutrino energy width is equivalent to that of the entangled recoil nucleus, which, in our case, is the upper limit on the measured energy width σ_ν,E = σ_N,E ≤ 2.9 eV. This limit was then converted into a momentum uncertainty using the relativistic energy–momentum relation p_ν = E_ν/c and σ_p = σ_E/c. The spatial uncertainty was extracted as σ_ν,x ≥ 34 nm at the 95% CL using the uncertainty principle. As our conservative method includes effects from sources of inhomogeneous broadening mentioned previously, the actual homogeneous broadening related to quantum uncertainty is expected to be markedly smaller than this limit. We also note that this method is equivalent to the approach prescribed in ref. ¹⁷ in which the uncertainty in energy comes from the inverse of the time for which wavepackets overlap at production, which depends on the velocities of the nuclei in the source. Our measured upper limit on the energy uncertainty is approximately 3–10 times larger than predictions from models based on atomic-scale localization and uncertainty in the time between atomic interactions in the reactor and ⁵¹Cr sources^1,3. Extending these theoretical models to make predictions for neutrinos emitted from nuclear decays in a sub-kelvin crystal lattice, as in the BeEST experiment, is beyond the scope of the present work.

For the second approach², the momentum widths of the recoiling nucleus and neutrino are equivalent, that is, σ_ν,p = σ_Li,p ≤ 16 keV c^–1, and the uncertainty principle again gives the spatial width. Under this approach, we determine a lower limit on the spatial width of the neutrino of σ_ν,x = σ_N,x ≥ 6.2 pm at the 95% CL (Fig. 3a). As the two methods described above yield markedly different results for the widths of the neutrino wavepackets (details of which are discussed extensively in the literature^1,2,3,4,17), we do not claim the validity of one over the other here. We instead use the more conservative of the two to set our reported lower limits. The result is equivalent to combining the momentum and energy uncertainties as proposed in refs. ^3,19,20.

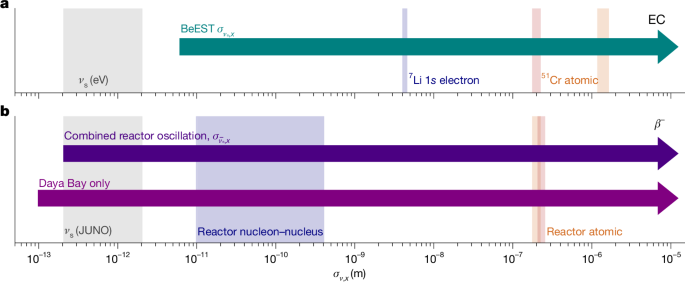
a,b, Comparison between experimental limits (horizontal bars) and theoretical estimates (vertical bars) for the spatial extent of a neutrino wavepacket created in EC sources (a) and reactor β⁻ sources (b). This plot encapsulates the current status of the spatial uncertainties of the neutrino wavepacket in both experiment and theory from the literature. For EC sources in a, the theoretical predictions are based on different scales of localization for ν_e. The blue band is based on the conservation of momentum and subatomic localization determined by the momentum uncertainty of the ⁷Li 1s electron orbital⁴. The red and orange bands are from two different predictions for atomic localization in a hot ⁵¹Cr source^1,3. For the reactor sources in b, the experimental limits are indirectly inferred from various oscillation experiments^5,6. The blue band from 10 pm to 400 pm is the theoretical estimate based on nucleon-scale/nucleus-scale localization estimates². The orange and red bands are estimates based on localization by atomic interactions in the reactor local environment^1,3. The bands on the far left spanning both plots correspond to the scenario in which the widths of the neutrino wavepackets are preferred in electronvolt-scale ν_s model fits to data^28,29, and in the case of reactor sources, would also be the region that wavepacket separation would be visible in JUNO^6,30.

Establishing an experimental distinction between localization models, particularly for a purely weak-interaction-mediated transition under the emission of a neutrino, would be a quantitative test of scale decoupling in quantum field theory⁵⁵, and may perhaps be the most important application of this technique in the future. For example, if the localizing interactions in weak nuclear decay are at the subatomic scale because of internal degrees of freedom in the nucleus^2,4, the resulting wavepackets are expected to be several orders of magnitude smaller than if the decay product widths are set by atomic interactions^1,3 (Fig. 3). A measurement precise enough to distinguish between these scenarios is expected to apply to all nuclear-decay-based ν sources and could serve as a fundamental test of quantum mechanics.

To perform a measurement that can distinguish the above models, our presented technique requires improvements and refinement in both analysis and detection methods. Potential improvements could come from accounting for known sources of broadening and minimizing lattice effects with more uniform superconducting films. We have shown that this technique can be applied to nuclear recoils of EC decay sources, but it may be possible to adapt it for the precision measurements of nuclear recoils of β^± decay sources in STJs with the upcoming Superconducting Array for Low-Energy Radiation experiment at the Facility for Rare Isotope Beams. As nuclear β decay does not involve the capture of an orbital electron in the initial state, it could enable a direct comparison with β⁻ sources and shed light on some of the fundamental questions on localization posed above (Fig. 3b).

The lower limit on the neutrino spatial width presented here does, however, provide potential insight into the suggestion that wavepacket separation may relieve tension in electronvolt-scale ν_s model fits with reactor experiments and the so-called ‘gallium anomaly’ in EC decay oscillation experiments. This improvement does, nevertheless, rely on the assumption that σ_ν,x varies neglibly between reactor β decay \({\overline{\nu }}_{e}\) sources and ⁵¹Cr ν_e EC sources²⁸. The same approach was also used to show that wavepacket separation could be one of the potential causes of the dampening-like effect that improves electronvolt-scale ν_s fits to global data²⁹. The impact of wavepacket separation in these fits is marked near the current experimental lower limit from the reactor data of σ_ν,x ≥ 210 fm but becomes indistinguishable from the plane-wave approximation at σ_ν,x ≥ 2 pm (ref. ²⁸). Our limit of σ_ν,x ≥ 6.2 pm for an EC source, as shown in Fig. 3a along with the region favoured by ν_s models, disfavours wavepacket separation as the correct explanation for this anomaly, unless the reactor and ⁵¹Cr sources both produce ν much more localized than the BeEST ⁷Be source. The region preferred by ν_s models also corresponds to the sensitivity region of JUNO^6,30. However, JUNO will detect \({\overline{\nu }}_{e}\) from nuclear reactors, and how this relates to an EC measurement depends on environmental factors and the scale of localization^1,2,3,4.

In summary, we present a new experimental concept to measure the low-energy recoiling daughter system from highly localized EC decaying rare isotopes inside superconducting sensors. Using this method, we have been able to directly constrain the quantum localization scale of the final-state system in electroweak nuclear decay. Here we report a conservative upper limit of the energy width of the recoiling ⁷Li system from the EC decay of ⁷Be in a tantalum matrix at 0.1 K of σ_N,E ≤ 2.9 eV (95% CL). This corresponds to a limit on the spatial width of the ⁷Li nuclear recoil of σ_N,x ≥ 6.2 pm, which suggests that the localization of the final-state system occurs at a scale much larger than the nucleus in which the interaction occurs. From this measurement, we also set the same limit on the spatial width of the entangled neutrino, σ_ν,x ≥ 6.2 pm, to our knowledge the first such limit on any EC ν_e source thus far and the first limit set by the direct measurements of the ν source. This limit disfavours wavepacket separation as the cause of dampening preferred by electronvolt-scale ν_s model fits to anomalous data. With planned future improvements to the analysis and detectors, this technique may be able to distinguish between competing models of localization in weak nuclear decays, and may provide a fundamental test of quantum mechanics.