Dental evidence for extended growth in early Homo from Dmanisi

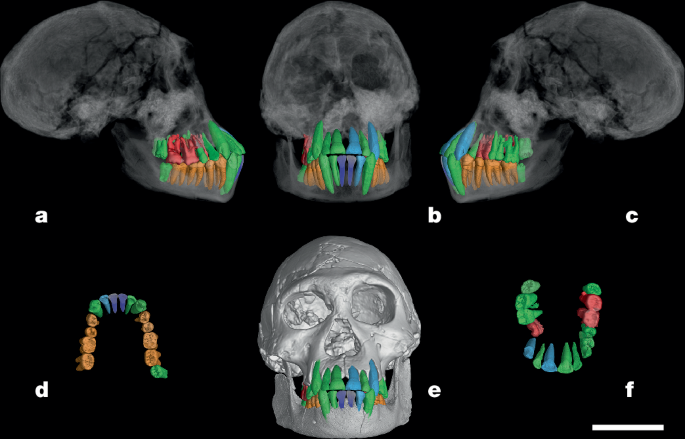
Propagation phase-contrast synchrotron microtomography

Cranium D2700 and associated mandible D2735 were scanned at the ID19 beamline of the European Synchrotron Radiation Facility, Grenoble, France. Volume scans were performed using PPC-SRµCT. For general dental morphological assessment, as well as for the counting of Andresen lines, two configurations were used in two successive series of experiments. The first used voxel sizes of 4.89 µm with a monochromatic beam set at 51 keV to take advantage of the gadolinium K-edge of the 5-μm-thick gadox scintillator. The second series took place a few years later, after substantial technical evolution of the ID19 beamline, and was performed using a 5.06-µm voxel size using a polychromatic beam whose spectrum was shaped with a tungsten filter to isolate a peak between the K-edge of the lutecium from the lutecium–aluminium–garnet–(cerium) scintillator (63.3 keV) and the K-edge of the tungsten filter (69.5 keV). This combination enabled much faster scans than with the monochromatic setup due to the higher flux and higher energy, and with perfect beam stability and improved coherence, resulting in much better phase-contrast imaging. In both cases, the propagation distance was 5,000 mm and the scans were performed in half-acquisition to almost double the field of view of the detector. In addition, scans for daily periodicity assessment were performed at 0.75 μm at 65 KeV, also in half-acquisition. All scanning parameters are listed in Supplementary Data 1, and a comparison of the monochromatic and polychromatic results is presented in Extended Data Fig. 7.

Acquisition of dental developmental data

Determination of developmental planes

The dental development was tracked based on the PPC-SRµCT data of at least one tooth of each type (Supplementary Data 1). Using the software VGStudio Max v.3.4 and later (Volume Graphics GmbH), virtual cross-sections through the teeth were generated to optimally visualize the microstructural growth lines in the enamel (Retzius lines) and dentin (Andresen lines). The optimum cross-sectional plane, named the developmental plane⁵⁵, was defined as the plane passing through a given dentin horn and the top of the underlying pulp cavity. The plane’s x axis was oriented in the bucco-lingual direction and its y axis along the tooth’s longitudinal axis. The plane’s thickness (z-axis depth) was set to values between 100 and 150 µm. The use of these ‘thick sections’ enhanced the visibility of the developmental microstructures in the tooth⁶.

Reconstruction of worn cusp tips

Several teeth of the Dmanisi individual had undergone substantial wear since eruption, as evidenced by missing enamel cusp tips and dentin horn tips (Extended Data Fig. 3). Because the most relevant microstructural developmental information in the teeth of this specimen came from the dentin (see ‘Daily secretion rate’), we focused on reconstructing the relatively small missing apical volumes of the dentin horns (Extended Data Fig. 3), using better-preserved teeth as guides. This process was repeated in several independent sessions. The reconstruction uncertainty inherent in this inference procedure is reflected in the calculations of the dental developmental timing and the age at death (Supplementary Data 1).

Matching of stress markers across the entire dentition

The general sequence of tooth development is defined by the order in which teeth initiate, emerge and complete their growth. Episodes of major stress during an individual’s development tend to affect the dentition as a whole, resulting in growth discontinuities, which become manifest as stress lines on the outer crown surface and in the microscopic record of enamel and dentin growth lines. A given stress event leaves its mark on all teeth that are still in the enamel and/or dentin deposition phase at this moment. This property is an important prerequisite for placing the development of the individual teeth in a common time frame (Extended Data Fig. 2). In the Dmanisi dentition, six major stress events could be identified (numbered 1 to 6 in Supplementary Data 1 and assigned different colours in Fig. 2). The matching of these stress lines across all teeth was ensured by cross-correlation between the Retzius and Andresen lines, and was further verified by comparison with the three-dimensional topology of the enamelo-dentin junction as well as the hypoplasias of the root surface (Extended Data Fig. 4) and lateral enamel surface (Extended Data Figs. 5 and 6), following the methodology proposed in ref. ⁵⁶.

Long-period duration

The deposition of dental enamel and dentin exhibit both short-term (daily) and long-term (several days) periodicities. In virtual PPC-SRµCT cross-sections, the daily incremental growth structures are visible as cross-striations (or laminations) in the enamel (with a size of about 1–3 μm)⁶. Typically, the cross-striations are most visible in the relatively flat areas of the mid-lateral cuspal enamel. In enamel, the long-period structures are visible as Retzius lines (size of about 30–40 μm, measured along the enamel prisms). The number of daily increments between consecutive Retzius lines gives the value of the long-period line periodicity. This is a property that varies between individuals of a given species (for example, in humans, between 5 and 12 days^28,57), but can also sometimes vary within a given individual, despite general consensus to the contrary. For example, in a modern human sample of n = 40, 18 individuals (45%) showed variation in long periodicity, with longer duration in the anterior than the posterior dentition⁵⁷.

To determine the actual long-periodicity of the D2700/D2735 dentition, scans of the enamel at a voxel resolution of 0.72 µm were performed on the URP4, URC, ULM3 and LRM3, and the cross-striations were directly traced and counted between the long-period lines (Supplementary Data 1 and 2 and Extended Data Fig. 2). Each tooth was evaluated by two independent observers, and 178 independent measurements were taken all over the teeth. Owing to strong artefacts in the data and the non-perfect preservation of the specimens, periodicities of between 3 and 9 days were observed. The 6-day periodicity represented 41% of the measurements, the 5-day periodicity 31% and the other periodicities 28%. Given the non-normal distribution of these values (Supplementary Data 2) (Kolmogorov–Smirnov test: F = 0.2465, P < 9.1693 × 10^−10), a Wilcoxon test was performed for comparison with expected periodicities of 5, 6 and 7 days, respectively. The only match between the expected and actual data was observed for the 6-day periodicity (P < 0.23), while the results for 5 (8.51 × 10^−9) and 7 days (7.31 ×  10^−10) suggest significant differences between the expected and observed distributions. Our data show no evidence of an antero-posterior gradient in the long-period duration⁵⁷. We thus used the 6-day periodicity to reconstruct the developmental profiles of all teeth by counting the Retzius and/or Andresen lines, and multiplying the total number of lines by the long-period duration.

Daily secretion rate

In the D2700/D2735 specimen, the long-period lines in the cuspal enamel (Retzius lines) were barely visible at the available voxel resolution (4.89 and 5.06 µm), which prevented direct measurements of the daily enamel secretion rate. However, the D2700/D2735 teeth exhibit exceptionally well-preserved dentin microstructures, which permitted an inference of the daily secretion rates by counting the Andresen lines in those areas where they were clearly visible. Specifically, the daily secretion rate of dentin was evaluated as follows. The length (L, in microns) of a given well-preserved primary dentin tubule was measured (secondary dentin tends to show compression of the Andresen line patterns), and the number, A, of the Andresen line intervals along the tubule was counted. The daily secretion rate (S, in microns per day) was then evaluated as S = L/(A × P), where P is the periodicity in days (Extended Data Fig. 2). The resulting value of 4.28 μm was high compared to humans and chimpanzees^30,58, but was cross-validated by two observers, and consistent results were obtained when the different sources of information were combined. Finally, this information was combined with the Andresen line counts and the cross-matched stress markers—visible in both the enamel and the dentin—to reconstruct the absolute developmental chronology of the whole dentition (Supplementary Data 1).

Dental eruption time

The time of dental eruption was estimated from the maximum extension rate of the root, termed the root growth spurt^32,58 (Extended Data Fig. 2). The method used to determine the root extension rates was adapted from previous studies^58,59 and proceeded as follows. Along the cemento-dentin junction, a first 200-μm-diameter circle was placed at the cervix and a second was aligned at the junction between the cemento-dentin junction and the Andresen line, passing tangentially on the side of the first circle (Extended Data Fig. 2). This procedure was repeated all along the length of the root. The greatest distance along the external surface of the root between the two circles marked the maximum extension rate, that is, the root growth spurt.

In great apes, the root growth spurt coincides with the period of dental eruption, whereas in modern humans, the growth spurt precedes eruption^32,58 (Supplementary Data 4). In the Dmanisi individual, therefore, the maximum rates of root growth should be regarded as minimum estimates of tooth eruption times. Furthermore, tooth eruption is a process that takes place over several months. We therefore represent the estimated eruption period as the time point of the maximum root extension rate ± 3 months (Fig. 2 and Extended Data Fig. 2). It should be noted that such a root growth spurt is visible in the third molars on the last third of the developing roots, while these teeth erupted quite some time before the death of the individual, as indicated by the light wear facets. This supports our basic assumption that the root growth spurt in this individual tends to coincide with the dental eruption.

Age at death and dental ontogenetic trajectory

Synopsis of dental development

The time-calibrated microstructural growth data of the tooth crowns and roots permitted reconstruction of the complete sequence of dental development throughout the life of D2700/D2735, from the initiation to completion of each tooth. The resulting diagram is visualized in Fig. 2 and the underlying data are presented in Supplementary Data 2. These data were used to determine the age at death of the individual, as well as its longitudinal dental ontogenetic trajectory.

Age at death

The age at death corresponds to the total time of dental development, from the crown initiation of the first molars (estimated from reconstructions of their worn cusp tips) to the last deposited dentin surface in the roots of the third molars. Because the M1 neonatal line was lost through dental wear, Dmanisi’s M1 crown initiation age had to be estimated from comparative data. In humans, the M1 initiation range is from approximately 0.15 years before to approximately 0.03 years after birth, that is, −0.06 ± 0.09 years (refs. ^60,61). With the M1 initiation set to birth (0 years), two independent analyses, performed by P.T. and V.B., yielded an age at death of 11.42 ± 0.56 years (Supplementary Data 1). Adding to this number the M1 initiation range yielded the following upper and lower bounds for the age at death of Dmanisi: −0.15 + 11.42 − 0.56 = 10.71 and 0.03 + 11.42 + 0.56 = 12.01 years, which together yield 11.36 ± 0.65 years.

Estimating the age at dental maturity

Dental maturity is reached with the root closure of the third molars. The time to root closure in the D2700/D2735 M3s was estimated by comparison with the homologous complete M2 roots. The M3 roots were scaled down to match the size of the M2 roots. Following the M2 dentin tubules, the distance from the open end of the M3 root to the closed M2 root tip was measured. Scaling this distance up to the original M3 size, and assuming an average dentin secretion rate of 4.28 μm, gives 469–561 days (about 1.3–1.5 years) to M3 root completion. The lower and upper bounds for the age at dental maturity are 10.71 + 1.3 = 12.0 years and 12.01 + 1.5 = 13.5 years.

Visualization of the ontogenetic trajectory

The ontogenetic trajectory was visualized using a video capturing the reconstructed developmental stages of each tooth at 6-month intervals, from birth to death, in the form of developmental plane sections (Supplementary Video 1). A second video (Supplementary Video 2) shows the same data in the form of virtual pseudo-orthopantomograms, which can be compared, to some extent, with clinical radiographic orthopantomograms.

The videos were constructed from consecutive virtual snapshots taken at specific time points along the ontogenetic trajectory as follows. Each tooth’s developmental plane (as defined above) was used as a base image. On each image, we first annotated the stress lines (Fig. 2 and Extended Data Figs. 4–6), which served as absolute time marks. Using the dentin and enamel extension rates as relative measurements of time, we then interpolated the locations of the Andresen and Retzius lines every 6 months, from birth to death. Then, working backwards from the complete developmental plane, dental material was removed virtually, and the resulting images were saved as snapshots for each 6-month step. The videos were recomposed from the single-tooth image sequences as follows. For each series (upper and lower dentition), two reference lines were defined to determine the position of the teeth before and after emergence, respectively. Pre-emergence teeth were positioned with their distal-most part at the pre-emergence line, while post-emergence teeth were positioned with their proximal-most part (crown cusps) at the post-emergence line. Finally, the images of all the teeth for each time step were merged together, and the timed snapshots were combined to form a video. The effects of tooth wear were not simulated in these videos because it would have been too difficult to make accurate interpolations of this process, so the crowns are shown with reconstructed cusp tips (recognizable by them having higher density than the preserved enamel), and the actual tooth wear is only shown at the time of death.

Ontogenetic trajectory of the dentition

The long dental development record of D2700/D2735 up to the subadult stage (well-developed, but open, M3 roots) allowed an almost complete dental ontogenetic trajectory to be reconstructed. The recovery of an individual longitudinal trajectory of 11.4 years is unique for fossil hominins, and even for individuals of living species, where trajectories are typically obtained by combining data from ontogenetic series rather than longitudinal ontogenetic samples. A scoring system developed previously¹³ that makes it possible to compare virtual sections with radiographic surveys was used to determine the overall maturation state of the entire dentition at 6-month intervals, from birth to death. Specifically, the maturation state of each tooth (I1, …, M3) was scored at each time step, following the procedures described in ref. ¹³. The resulting DMSs (DMS_I1, …, DMS_M3) obtained from the virtual histology sections were then converted into equivalent clinical radiographic scores¹³ to permit direct comparisons with the radiographic data from the literature. Finally, at each time step, the DMSs of all the teeth were summed to obtain a total DMS (standardized to 100%). The resulting longitudinal ontogenetic trajectories of D2700/D2735 are visualized in Fig. 3 (DMS_I1, …, DMS_M3) and Fig. 4 (total DMS).

Comparative data

Comparative data on the developing dentition of known-age chimpanzees and humans were collected from the literature (Supplementary Data 4). The data for chimpanzees were from refs. ^35,36,62 and comprise all eight permanent tooth types (I1–M3). The known-age human dataset was combined from studies examining all eight teeth (I1–M3) (refs. ^40,63,64,65) or seven teeth (I1–M2) (refs. ^{63,65,66,67,68,69,70,71,72,73}). Comparative data for Pan paniscus came from ref. ⁷⁴. The data for Gorilla and Pongo were acquired from medical CT scans of collection specimens (Supplementary Data 4 and ref. ³⁸). Data on the dental ontogeny of fossil hominin specimens representing Australopithecus, Paranthropus and early Homo were collected from the literature^{9,12,13,48,75,76,77}. Comparative data on M1 eruption were from refs. ^32,34,38,78.

The primary data used in our comparative analyses were the DMSs of each tooth type (DMS_I1, …, DMS_M3), and of the dentition as a whole (total DMS = sum of DMS_I1, …, DMS_M3). The included studies were based on different dental-imaging methods (dental radiography, CT, synchrotron-based tomography) and used different DMS systems, which required prior calibration and standardization^13,79. As a common reference, we used the Demirjian DMS system⁶⁶, which subdivides dental maturation into eight stages (four stages each for crown and root formation). The calibration schemes used to convert the 10-, 12- and 14-stage DMS systems to the 8-stage Demirjian system are listed in Supplementary Data 4. All calibrated data were standardized to total DMS = 100% for a fully formed dentition. Consistency checks to ensure the comparability of the data are described in detail further below. These checks show that, after calibration, the potential residual bias due to the use of different imaging methods and different scoring systems did not exceed the natural variation found in 1-year age bins of chimpanzees and/or humans.

Data analysis

We analysed the ontogeny of the dentition as a whole (hereafter referred to as the dental ontogeny) and focused on two aspects, the ontogenetic pattern and rate.

The ontogenetic pattern of an individual’s dentition was given by the set of DMSs of the eight tooth types (DMS_I1, …, DMS_M3). For a sample of n specimens, which resulted in an n × 8 DMS matrix. To analyse these multivariate data, we used the approach proposed in ref. ³⁵. Using the software JMP v.15.2 (SAS Institute Inc.), a PCA was performed on the n × 8 DMS matrix, resulting in eight PCs that accounted for the largest to smallest proportions of the total variance in the sample. Typically, the first few components of a PCA comprise a large proportion of the total variance in the sample, which permits visualization of the relevant patterns of variation in the sample in a low-dimensional subspace.

The DMS-PCA method, as we call it here, made it possible to document variation along and across ontogenetic trajectories and to identify taxon-specific differences between the maturation states of the different tooth types relative to each other (Fig. 3b). Ontogenetic trajectories start with no teeth (0,0,0,0,0,0,0,0) and end with all eight fully formed teeth (1,1,1,1,1,1,1,1), so that, in multivariate space, all trajectories have the same starting point, then diverge, finally converging again to a single end point. Therefore, taxon discrimination is best in the middle of the trajectory.

In our analysis of Fig. 3b, PC1 accounted for variation along the ontogenetic trajectories and was essentially equivalent to the total DMS (PC1 = −16.105 + 0.284 × DMS; R²â€‰= 0.9999; P < 0.0001), as already noted in ref. ³⁵, with PC2 accounting for taxon-specific differences between trajectories. It is important to note that individual age was not part of the DMS-PCA, but served as an external variable to time-stamp taxon-specific trajectories through multivariate space. Therefore, specimens of unknown individual age, such as wild-lived great apes and many hominin fossils, can also be included in the analysis.

While the DMS-PCA method provided a detailed and comprehensive picture of dental ontogenetic patterns, we noted that alternative statistical methods have been used to reach similar conclusions regarding the differences between great ape, human and fossil hominin patterns of dental ontogeny^48,80.

Ontogenetic rate

To quantify the rate at which the dentition of a given individual or taxon developed, the total DMS (sum of DMS_I1, …, DMS_M3) was plotted against age. The resulting total DMS trajectories are visualized in Fig. 4a. Furthermore, to summarize the differences between taxon-specific trajectories, we evaluated instantaneous ontogenetic rates = (DMS(t_i) − DMS(t_i−Δt))/Δt, with Δt = 0.5 years (Fig. 4b). Using MS Excel v.16.16.27 software (Microsoft Office), a moving-average function, comprising time steps t_i−Δt, t_i and t_i+Δt, with Δt = 0.5 years, was then applied to level out, to some extent, the discontinuities resulting from the discrete nature of the scoring procedure (Supplementary Data 4). The instantaneous ontogenetic rates trajectory of chimpanzees was evaluated from the data in refs. ^35,36,62. The human instantaneous ontogenetic rate trajectories were evaluated per population from the maturation score data for eight teeth^{40,63,64,65,81} or seven teeth^{63,65,67,68,69,70,72,73}. The human DMS trajectories show a DGS, the timing of which varies between populations (6.3–8.5 years) (see main text and Extended Data Table 1). The upper range (8.5 years) largely coincides with M3 crown initiation, such that seven-teeth scoring schemes are unlikely to bias DGS estimates compared to eight-teeth scoring schemes.

Consistency checks

Influence of imaging methods and scoring schemes on dental maturation trajectories

To assess how different imaging methods and different scoring schemes affected the evaluation of the dental ontogenetic rate and pattern, we used data from two independent studies of dental ontogeny in Finnish children, here named Haavikko70 (ref. ⁴⁰) and Nyström07 (ref. ⁸²). The Haavikko70 data were based on a ten-stage DMS scheme, whereas the Nyström07 data used the eight-stage Demirjian scheme⁶⁶. To assess the effects of imaging methodology (traditional dental radiography versus tomography-based virtual histology), we recoded the Haavikko70 data from the original radiographic to histological (tomographic) scores, using the conversion scheme presented in ref. ¹³. To assess the effects of data standardization, we recoded the Haavikko70 data with the ten-to-eight-stage recoding scheme presented in Supplementary Data 4. The analyses presented in Extended Data Fig. 8a,b thus compare four datasets: Nyström07 eight-stage, Haavikko70 ten-stage, Haavikko70 eight-stage radiography and Haavikko70 eight-stage virtual histology. We evaluated the DMS-versus-age profiles (Extended Data Fig. 8a), and used a DMS-PCA to visualize the ontogenetic trajectories through multivariate space (Extended Data Fig. 8b). Then results show that the different scoring schemes yielded different DMS-versus-age profiles, but that standardization led to good correspondence between the two datasets. As noted earlier^12,13,83, the uncalibrated data from the virtual histology yielded total DMS-versus-age profiles that were consistently more advanced than the radiography-based profiles. Interestingly, however, in the DMS-PCA space, all four datasets (calibrated and uncalibrated) showed dental maturation trajectories that largely coincided, indicating that the DMS-PCA was robust to differences in imaging methods and scoring systems.

Dental maturation profiles and evaluation of the dentition growth spurt

To assess the influence of different DMS schemes on the evaluation of the DGS, we compared dental maturation data from three independent studies on Finnish children^40,67,82. These studies used different scoring schemes (ten-stage versus eight-stage), and different tooth arrays (including/excluding M3) (Extended Data Fig. 8c and Supplementary Data 4). Nevertheless, the DMS rate peaks were at similar locations along the age axis (Extended Data Fig. 8d), indicating that the evaluation of DGS was robust to differences in DMS scoring schemes.

Assessment of age-related variation in dental maturation patterns along taxon-specific ontogenetic trajectories

DMS-PCA was performed on known-age humans and chimpanzees. As shown in Extended Data Fig. 8e, there is intra-taxon overlap between the 1 s.d.-density ellipses around consecutive 1-year age groups. The natural variation within 1-year age groups along the trajectories tended to be greater than the potential bias remaining after calibration for different data acquisition and scoring methods (Extended Data Fig. 8b).

Reporting summary

Further information on research design is available in the Nature Portfolio Reporting Summary linked to this article.