Efficient conversion of syngas to linear α-olefins by phase-pure χ-Fe5C2

Materials

Raney iron precursor

Iron-aluminium alloy powder (50:50 by weight, Sigma-Aldrich) was added into an 8 mol l^−1 KOH (AR, Sinopharm Chemical Reagent) solution in a flask under stirring and heated to 70 °C to dissolve the aluminium in the alloy^36,37. Afterwards, K⁺ and \({{\rm{AlO}}}_{2}^{-}\) ions were removed by washing with deionized water (ten times) and ethanol (seven times). The iron powder sample was transferred by means of a sealable quartz tube into a glove box and subsequently dried in an argon flow at room temperature for 6 h. The resulting porous iron powder was kept in a container with a seal in a glove box. Before loading the sample in appropriate in situ cells (XRD and Mössbauer spectroscopy characterization) or a stainless-steel reactor for FT activity measurements, the sample was passivated in a flow of 1% O₂ in helium at room temperature for 20 h.

Manganese-Raney iron precursor

Manganese (0.5–12.5 wt%) was added to the Raney iron precursor (directly after removal of aluminium) by wet impregnation using a Mn(NO₃)₂ solution. The sample was then dried in a vacuum oven at room temperature for 12 h.

Potassium-Raney iron precursor (Extended Data Fig. 3c)

Potassium (1 wt%) was added to the Raney iron precursor (directly after removal of aluminium) by wet impregnation using a KNO₃ solution. The sample was then dried in a vacuum oven at room temperature for 12 h.

Characterization

In situ X-ray diffraction (in situ XRD) was carried out on a Rigaku D/max-2600/PC instrument equipped with a D/teX ultrahigh-speed detector and scintillation counter. The X-ray generator consisted of a copper rotating anode with a maximum power of 9 kW. All measurements were carried out at 40 mA and 40 kV. In situ XRD patterns were recorded in an Anton Par XRK-900 cell equipped with a CO/H₂/Ar gas inlet system.

Environmental TEM (ETEM) images were recorded in an aberration-corrected FEI Titan ETEM G2 instrument at an acceleration voltage of 300 kV (ref. ³⁸). Syngas was introduced for 1 h under 1,200 Pa at 320 °C as a pretreatment. The carburization temperature was then raised to 350 °C in about 0.5 h, followed by monitoring the sample continuously.

In situ Mössbauer spectroscopy was carried out in an in situ high-pressure cell suitable for Mössbauer spectroscopy³⁹. Transmission ⁵⁷Fe Mössbauer spectra were collected at −153 °C with a sinusoidal velocity spectrometer using a ⁵⁷Co(Rh) source. The source and the sample were kept at the same temperature during the measurements. Mosswinn 4.0 software was used for spectra fitting⁴⁰.

Catalytic activity measurements

In situ carburization of χ-Fe₅C₂ and Mn-χ-Fe₅C₂ and FTLAO catalytic activity measurements

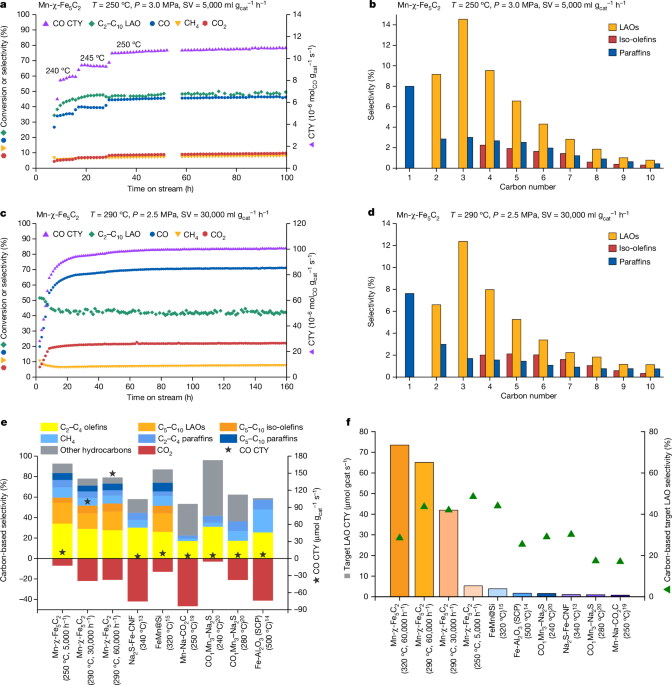
A 75 mg amount of Raney iron precursor or manganese/Raney iron precursor, the latter also containing 75 mg of Raney iron, was diluted with 1,500 mg silicon carbide and loaded into a stainless-steel tubular fixed-bed reactor with an external diameter of 14.5 mm, internal diameter of 9 mm, length of 305 mm and total internal volume of 20 ml. An exception was the test in Table 1, row 1, for which 225 mg Raney iron of a manganese-Raney iron precursor was diluted with 4,500 mg silicon carbide to achieve a low SV of 5,000 ml \({{\rm{g}}}_{{\rm{cat}}}^{-1}\,{{\rm{h}}}^{-1}\). The catalyst precursor was in situ carburized in a syngas flow (H₂/CO/He = 100/3.2/21.8) while increasing the temperature to 350 °C at a rate of 1 °C min^−1, followed by a dwell of 6 h at ambient pressure and an SV of 75,000 ml \({{\rm{g}}}_{{\rm{cat}}}^{-1}\,{{\rm{h}}}^{-1}\). After cooling the reactor to 250 °C (240 °C for the test in Table 1, row 3), the reactor feed was switched to the feed mixture and the reactor pressure was increased to the desired value. The start of the reaction was defined as the time at which these conditions were reached. The reactor was then ramped to the indicated temperature.

For the comparison between χ-Fe₅C₂ and Mn-χ-Fe₅C₂ (Table 1, rows 1 and 2), the reaction conditions were as follows: a feed mixture SV of H₂/CO/He = 12,000/8,000/8,000 ml \({{\rm{g}}}_{{\rm{cat}}}^{-1}\,{{\rm{h}}}^{-1}\)), a reaction pressure of 2.3 MPa and a reaction temperature of 250 °C. For the evaluation of the Mn-χ-Fe₅C₂ catalyst under different temperatures and pressures (Fig. 1a–f and rows 3–6 of Table 1), the reaction conditions were as follows: a feed mixture of H₂/CO/Ar (internal standard) = 1.5/0.95/0.05 at a total SV of 5,000, 30,000 or 60,000 ml \({{\rm{g}}}_{{\rm{cat}}}^{-1}\,{{\rm{h}}}^{-1}\), a reaction pressure of either 2.5 or 3.0 MPa and a reaction temperature of 250, 290 or 320 °C (heating rate 0.1 °C min^−1). For the evaluation of the Mn-χ-Fe₅C₂ catalyst in Extended Data Table 2, the reaction conditions were as follows: a feed mixture SV of H₂/CO = 1.5 at a total SV of 30,000, 60,000, 75,000 or 90,000 ml \({{\rm{g}}}_{{\rm{cat}}}^{-1}\,{{\rm{h}}}^{-1}\) with no inert gas added, a reaction pressure of 2.5 MPa and a reaction temperature of 250, 270, 290 or 310 °C (heating rate 0.1 °C min^−1). For the evaluation of Mn-χ-Fe₅C₂ (Extended Data Fig. 5a) and K-χ-Fe₅C₂ (Extended Data Fig. 3c), the reaction conditions were as follows: a feed mixture SV of H₂/CO/He = 36,000/24,000/24,000 ml \({{\rm{g}}}_{{\rm{cat}}}^{-1}\,{{\rm{h}}}^{-1}\) or 72,000/48,000/48,000 ml \({{\rm{g}}}_{{\rm{cat}}}^{-1}\,{{\rm{h}}}^{-1}\) and a reaction pressure at 2.3 MPa. The reaction temperature was 315 or 325 °C for these measurements (heating rate 0.5 °C min^−1). For the long-term test for Mn-χ-Fe₅C₂ (Extended Data Fig. 5c), the reaction conditions were as follows: a feed mixture SV of H₂/CO/He = 60,000/40,000/40,000 ml \({{\rm{g}}}_{{\rm{cat}}}^{-1}\,{{\rm{h}}}^{-1}\) and a reaction pressure at 2.3 MPa. We used a low ramp rate of 2 °C h^−1 to avoid overheating issues due to the exothermicity of the reaction. Therefore, it took 35 h to raise the temperature from 250 °C to 320 °C.

The effluent gas flow was analysed by an online Agilent 7890 gas chromatograph equipped with two thermal conductivity detectors and one flame ionization detector. Each result was acquired from a single experiment. All of the SVs are calculated on the basis of the weight of the Raney iron catalyst precursor without a promoter or silicon carbide diluent.

The CO conversion and product selectivity were calculated as below.

The CO conversion (\({X}_{{\rm{CO}}}\)) was calculated by

The CO₂ selectivity (\({S}_{{{\rm{CO}}}_{2}}\)) was calculated by

The carbon-based hydrocarbon selectivity (\({S}_{{{\rm{C}}}_{x}{{\rm{H}}}_{y}}\)) was calculated by

The indicated parameters represent the inlet and outlet molar flows determined.

The CO catalyst time yield (\({{\rm{CTY}}}_{{\rm{CO}}}\)) was calculated by

The carbon-based hydrocarbon catalyst time yield (\({{\rm{CTY}}}_{{{\rm{C}}}_{x}{{\rm{H}}}_{y}}\)) was calculated by

The experimental chain-growth probability was calculated by the ASF distribution as follows

in which n is the number of carbon atoms in a particular hydrocarbon product, W_n is the weight fraction of a product with n number of carbon atoms and α is the chain-growth probability.

Theoretical modelling

Density functional theory calculations

Density functional theory (DFT) calculations were carried out to obtain the energetics for elementary reaction steps relevant to the FT reaction on χ-Fe₅C₂. All spin-polarized DFT calculations were conducted using the projector augmented-wave method and the Perdew–Burke–Ernzerhof functional, as implemented in the Vienna ab initio simulation package code. Solutions of the Kohn–Sham equations were obtained using a basis set of plane waves with a cutoff energy of 400 eV. Sampling of the Brillouin zone was carried out using a 5 × 5 × 1 k-point mesh. Higher cutoff energies or a finer Brillouin zone sampling did not lead to substantial energy differences. All atoms were allowed to relax during the optimization of the empty surfaces. We used a 2 × 2 × 1 unit cell for the (100) surface, containing 80 iron and 32 carbon atoms, with a layer thickness of 10.31 Å. A vacuum layer of 15 Å was added perpendicular to the surface to avoid spurious interactions between neighbouring images. Adsorption of atoms and molecules was carried out on the top side of the slab, whereas the lower half was frozen. A dipole correction was carried out for all adsorbed states. Further technical details such as Vienna ab initio simulation package settings for these iron carbide calculations are described elsewhere²³. The adsorption energies of the gas-phase molecules were determined by subtracting the energies of the empty surface and the free adsorbate from the adsorbed state. The energy of the adsorbate in the gas phase was obtained by placing a molecule at the centre of a 10 × 10 × 10 ų unit cell, using the Γ-point for k-point sampling. Transition states were acquired using the nudged elastic band method⁴¹. A frequency analysis was carried out to confirm that all transition geometries correspond to a first-order saddle point on the potential energy surface with an imaginary frequency in the direction of the reaction coordinate. The corresponding normal-mode vibrations were also used to calculate the zero-point energy correction. We also corrected the barriers for the migration of fragments after dissociation by considering the energy difference of the geometry directly after dissociation and their most stable adsorption positions at infinite distance.

We carried out DFT calculations to determine the energetics of elementary reaction steps of the conversion of synthesis gas into hydrocarbons (methane, olefins and paraffins) and CO₂ and H₂O. The energy barriers and their corresponding pre-exponential factors are listed in Extended Data Table 1. The (100) surface of χ-Fe₅C₂ was selected, because this surface is a stable surface termination of Hägg carbide and also allows for facile C–O bond dissociation, which is an essential step in the FT reaction⁴². Extended Data Table 1 shows forward and backward activation energies and the corresponding pre-exponential factors for the consecutive hydrogenation steps of adsorbed carbon to methane and the removal of oxygen as H₂O and CO₂. Extended Data Table 1 shows forward and backward activation energies and the corresponding pre-exponential factors for the C–C coupling reactions and the hydrogenation to ethylene and ethane.

Microkinetics modelling

For the construction of the microkinetic model of the FT reaction, differential equations for all reaction intermediates on the catalytic surface were constructed using the rate constants of all considered elementary reaction steps. Herein, we assumed that all adsorbates occupy one active site. For adsorption, we assumed that the adsorbate loses one translational degree of freedom in the transition state with respect to the initial state. For desorption, we assumed that the species gains two translational degrees of freedom and three rotational degrees of freedom in the transition state with respect to the initial state. From these two assumptions, the rate of adsorption and desorption are as follows:

Herein, \({k}_{{\rm{ads}}}\) is the rate constant for the adsorption of the adsorbate, P is the pressure in pascals, A is surface area in square metres, m is the mass of the reactant in kilograms, k_B is the Boltzmann constant in joules per kelvin, T is the temperature in kelvin, \({k}_{{\rm{des}}}\) is the rate constant for the desorption of the adsorbate, h is the Planck constant in joules multiplied by seconds, \(\sigma \) is the symmetry number, \({\theta }_{{\rm{rot}}}\) the rotational temperature in kelvin, E_des is the desorption energy in joules per mole, and R is the gas constant in joules per kelvin per mole.

The rate constant (k) of an elementary reaction step was determined using the Eyring equation, which is defined as follows:

in which \({E}_{{\rm{act}}}\) is activation energy in joules per mole, k_B the Boltzmann constant, T the temperature in kelvin, and \(v\) the pre-exponential factor in the unit of per second. Pre-exponential factors for the forward and backward reactions can be obtained using:

and

in which \({v}_{{\rm{forward}}}\) and \({v}_{{\rm{backward}}}\) refer to the pre-exponential factors for the forward and the backward reaction, respectively, q_vib is the vibrational partition function of the initial state (IS) and the transition state (TS), and h is Planck’s constant.

All microkinetic simulations were carried out using the MKMCXX software suite⁴³. The set of differential equations were time-integrated using the backward differentiation formula method until a steady-state solution was obtained. From the steady-state coverages, the rates of the individual elementary reactions steps were obtained using a flux analysis, as implemented in the MKMCXX software. To mimic experimental conditions, the pressure was set to 0.1 MPa over a temperature range between 510 K and 545 K. We adopted a continuously stirred tank reactor with ideal mixing using an SV chosen to obtain differential conditions over the whole temperature range. The H₂/CO ratio was kept constant at 2:1. Chain growth was considered by involving coupling of two CH_x adsorbates. Chain growth for hydrocarbon chains up to 20 carbon atoms was considered by treating the growing chain as CR, in which R = alkyl chain and considering that barriers for chain growth are independent of chain length. Adsorption energies of C₂ and C₃ intermediates were taken into account explicitly, whereas those of hydrocarbon fragments with more than three carbon atoms were taken to be equal to those of C₃ intermediates. Proper entropy corrections were made depending on the chain length of the hydrocarbons.

The chain-growth probability was determined from the ASF distribution by considering hydrocarbon products containing 1–20 carbon atoms:

Herein, the chain-growth probability is defined as the rate of propagation (\({r}_{{\rm{p}}}\)) over the sum of the rates of propagation and termination (\({r}_{{\rm{t}}}\)). \({F}_{{{\rm{C}}}_{n}}^{{\rm{out}}}\) corresponds to the flow rate of \({C}_{n}\) in the experiment. This involved simulating the corresponding chain-growth probability (α) and C₂ selectivity within the ASF distribution shown in Extended Data Fig. 2.

Results of microkinetic simulations

Extended Data Fig. 1a shows the CO conversion rate and formation rates of CH₄ (C₁) and longer hydrocarbons (C₂₊) for the (100) surface of χ-Fe₅C₂ as a function of temperature. As expected, the rates exponentially increase with temperature owing to the Arrhenius dependence of the reaction rate constants. The CO conversion rate is in the same range as experimentally observed for iron carbide catalysts^42,44. The selectivity towards CH₄ is lower than the total selectivity towards other hydrocarbons, which is important because CH₄ has a much lower value than higher hydrocarbons. Extended Data Fig. 2a shows the hydrocarbon product distribution, which indicates that, except for C₁ and C₂, the longer hydrocarbons are statistically distributed according to the ASF theory. The parameter describing this distribution is the chain-growth probability and its temperature dependence is shown in Extended Data Fig. 2b. This parameter reflects the statistical nature of the growth process in which hydrocarbons can either grow by addition of a C₁ monomer or desorb as a product. The decrease with temperature shows that termination as products has a higher overall activation energy than chain growth. The value of about 0.5 is slightly lower than experimentally observed in our study. Extended Data Fig. 2c shows the simulated O/P ratio to be around 3.0 with a minor dependence on temperature. These data show that both olefins and paraffins are formed as primary products. The O/P values are in the same range as experimentally observed, namely about 1.5 for the unpromoted catalyst and about 3.6 for the manganese-promoted catalyst. Extended Data Fig. 1b demonstrates that oxygen atoms originating from CO dissociation are predominantly removed as H₂O, instead of CO₂. These findings provide an explanation for the low CO₂ selectivity observed in our experimental study in which a pure χ-Fe₅C₂ catalyst was used. In other studies in which the catalyst precursor is not completely converted to χ-Fe₅C₂, competing phases often include iron oxides, which are known as good catalysts for the (reverse) water–gas shift reaction.

The computational predictions in Extended Data Table 1 show that, on χ-Fe₅C₂, the overall barrier for H₂O formation (155 kJ mol^−1) is lower than the barrier for CO₂ formation (181 kJ mol^−1). This implies that H₂O formation is preferred on χ-Fe₅C₂ over CO₂ formation as the oxygen removal step. To verify this prediction based on the overall energy barrier under reaction conditions, we used microkinetic simulations based on DFT-based reaction energetics and found that oxygen removal reactions proceed primarily through the formation of H₂O (99.4% at 525 K) instead of CO₂ (0.6% at 525 K; Extended Data Fig. 1c). These simulations showing a very low CO₂ selectivity pertain to the zero-conversion limit and, thus, represent so-called primary CO₂ production. The microkinetic simulations also provide a deeper insight into the reaction mechanism including the interplay between the surface intermediates. In Extended Data Fig. 1d we show that, in addition to CO dissociation, oxygen removal and carbon hydrogenation (as part of the chain-growth mechanism) control the overall reaction rate. These findings are in keeping with the periodic trends predicted in previous work⁴³.

Rietveld refinement XRD patterns

The results of Rietveld refinement of the XRD patterns using the Fullprof software are shown in Extended Data Fig. 7g. Rietveld refinement confirmed the phase purity of χ-Fe₅C₂ (no substantial contribution of other phases) with a goodness of fit of χ²â€‰= 7.58%, R_p = 18.7%, R_wp = 15.3% and R_exp = 5.6%. The space group and lattice parameters listed in Extended Data Fig. 7g are in good agreement with published data for χ-Fe₅C₂ (ref. ⁴⁵). The atomic positions of the specific sites for the χ-Fe₅C₂ structure are listed in Extended Data Fig. 7h. Note that the data were acquired in an in situ XRD reaction chamber under a nitrogen flow after obtaining the χ-Fe₅C₂ particles through the described synthesis method.